Classical and Quantum Gravity

Interstellar is the first Hollywood movie to attempt depicting a black hole as it would actually be seen by somebody nearby. For this, our team at Double Negative Visual Effects, in collaboration with physicist Kip Thorne, developed a code called Double Negative Gravitational Renderer (DNGR) to solve the equations for ray-bundle (light-beam) propagation through the curved spacetime of a spinning (Kerr) black hole, and to render IMAX-quality, rapidly changing images. Our ray-bundle techniques were crucial for achieving IMAX-quality smoothness without flickering; and they differ from physicists' image-generation techniques (which generally rely on individual light rays rather than ray bundles), and also differ from techniques previously used in the film industry's CGI community. This paper has four purposes: (i) to describe DNGR for physicists and CGI practitioners, who may find interesting and useful some of our unconventional techniques. (ii) To present the equations we use, when the camera is in arbitrary motion at an arbitrary location near a Kerr black hole, for mapping light sources to camera images via elliptical ray bundles. (iii) To describe new insights, from DNGR, into gravitational lensing when the camera is near the spinning black hole, rather than far away as in almost all prior studies; we focus on the shapes, sizes and influence of caustics and critical curves, the creation and annihilation of stellar images, the pattern of multiple images, and the influence of almost-trapped light rays, and we find similar results to the more familiar case of a camera far from the hole. (iv) To describe how the images of the black hole Gargantua and its accretion disk, in the movie Interstellar, were generated with DNGR—including, especially, the influences of (a) colour changes due to doppler and gravitational frequency shifts, (b) intensity changes due to the frequency shifts, (c) simulated camera lens flare, and (d) decisions that the film makers made about these influences and about the Gargantua's spin, with the goal of producing images understandable for a mass audience. There are no new astrophysical insights in this accretion-disk section of the paper, but disk novices may find it pedagogically interesting, and movie buffs may find its discussions of Interstellar interesting.

Recently, we introduced a non-perturbative quantization of impulsive gravitational null initial data. In this note, we investigate an immediate physical implication of the model. One of the quantum numbers is the total luminosity carried to infinity. We show that a transition happens when the luminosity reaches the Planck power 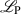$\mathscr{L}_{\mathrm{P}}$. Below 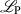$\mathscr{L}_{\mathrm{P}}$, the spectrum of the radiated power is discrete. Above the Planck power, the spectrum is continuous. A physical state that lies in the continuous spectrum consists of a superposition of kinematical states in which the shear is unbounded from above. We argue that such states are unphysical because they contain caustics that are in conflict with the falloff conditions at asymptotic infinity.

The theory of general relativity predicts the existence of closed time-like curves (CTCs), which theoretically would allow an observer to travel back in time and interact with their past self. This raises the question of whether this could create a grandfather paradox, in which the observer interacts in such a way to prevent their own time travel. Previous research has proposed a framework for deterministic, reversible, dynamics compatible with non-trivial time travel, where observers in distinct regions of spacetime can perform arbitrary local operations with no contradiction arising. However, only scenarios with up to three regions have been fully characterised, revealing only one type of process where the observers can verify to both be in the past and future of each other. Here we extend this characterisation to an arbitrary number of regions and find that there exist several inequivalent processes that can only arise due to non-trivial time travel. This supports the view that complex dynamics is possible in the presence of CTCs, compatible with free choice of local operations and free of inconsistencies.

We study the internal dynamics of a hypothetical spaceship traveling on a close timelike curve in an axially symmetric Universe. We choose the curve so that the generator of evolution in proper time is the angular momentum. Using Wigner's theorem, we prove that the energy levels internal to the spaceship must undergo spontaneous discretization. The level separation turns out to be finely tuned so that, after completing a roundtrip of the curve, all systems are back to their initial state. This implies, for example, that the memories of an observer inside the spaceship are necessarily erased by the end of the journey. More in general, if there is an increase in entropy, a Poincaré cycle will eventually reverse it by the end of the loop, forcing entropy to decrease back to its initial value. We show that such decrease in entropy is in agreement with the eigenstate thermalization hypothesis. The non-existence of time-travel paradoxes follows as a rigorous corollary of our analysis.

 The simplest ΛCDM model provides a good fit to a large span of cosmological data but harbors large areas of phenomenology and ignorance. With the improvement of the number and the accuracy of observations, discrepancies among key cosmological parameters of the model have emerged. The most statistically significant tension is the 4σ to 6σ disagreement between predictions of the Hubble constant, H₀, made by the early time probes in concert with the 'vanilla' ΛCDM cosmological model, and a number of late time, model-independent determinations of H₀ from local measurements of distances and redshifts. The high precision and consistency of the data at both ends present strong challenges to the possible solution space and demands a hypothesis with enough rigor to explain multiple observations—whether these invoke new physics, unexpected large-scale structures or multiple, unrelated errors. A thorough review of the problem including a discussion of recent Hubble constant estimates and a summary of the proposed theoretical solutions is presented here. We include more than 1000 references, indicating that the interest in this area has grown considerably just during the last few years. We classify the many proposals to resolve the tension in these categories: early dark energy, late dark energy, dark energy models with 6 degrees of freedom and their extensions, models with extra relativistic degrees of freedom, models with extra interactions, unified cosmologies, modified gravity, inflationary models, modified recombination history, physics of the critical phenomena, and alternative proposals. Some are formally successful, improving the fit to the data in light of their additional degrees of freedom, restoring agreement within 1–2σ between Planck 2018, using the cosmic microwave background power spectra data, baryon acoustic oscillations, Pantheon SN data, and R20, the latest SH0ES Team Riess, et al (2021 Astrophys. J.908 L6) measurement of the Hubble constant (H₀ = 73.2 ± 1.3 km s⁻¹ Mpc⁻¹ at 68% confidence level). However, there are many more unsuccessful models which leave the discrepancy well above the 3σ disagreement level. In many cases, reduced tension comes not simply from a change in the value of H₀ but also due to an increase in its uncertainty due to degeneracy with additional physics, complicating the picture and pointing to the need for additional probes. While no specific proposal makes a strong case for being highly likely or far better than all others, solutions involving early or dynamical dark energy, neutrino interactions, interacting cosmologies, primordial magnetic fields, and modified gravity provide the best options until a better alternative comes along.

We construct a (quantum mechanically) modified model for the Oppenheimer–Snyder collapse scenario where the exterior of the collapsing dust ball is a Hayward black hole spacetime and the interior is a dust Friedmann–Robertson–Walker cosmology. This interior cosmology is entirely determined by the junction conditions with the exterior black hole. It turns out to be non-singular, displaying a power-law contraction which precedes a de Sitter phase or, reversely, a power-law expansion followed by a de Sitter era. We demonstrate that cosmic inflation in the collapse setting is a mechanism that decelerates collapsing matter, thereby preventing singularity formation. We also analyse the global causal structure and the viability of the model.

The Laser Interferometer Space Antenna (LISA) mission is being developed by ESA with NASA participation. As it has recently passed the Mission Adoption milestone, models of the instruments and noise performance are becoming more detailed, and likewise prototype data analyses must as well. Assumptions such as Gaussianity, stationarity, and data continuity are unrealistic, and must be replaced with physically motivated data simulations, and data analysis methods adapted to accommodate such likely imperfections. To this end, the LISA Data Challenges have produced datasets featuring time-varying and unequal constellation armlength, and measurement artifacts including data interruptions and instrumental transients. In this work, we assess the impact of these data artifacts on the inference of galactic binary and massive black hole properties. Our analysis shows that the treatment of noise transients and gaps is necessary for effective parameter estimation, as they substantially corrupt the analysis if unmitigated. We find that straightforward mitigation techniques can significantly if imperfectly suppress artifacts. For the Galactic Binaries, mitigation of glitches was essentially total, while mitigations of the data gaps increased parameter uncertainty by approximately 10%. For the massive black hole binaries the particularly pernicious glitches resulted in a 30% uncertainty increase after mitigations, while the data gaps can increase parameter uncertainty by up to several times. Critically, this underlines the importance of early detection of transient gravitational waves to ensure they are protected from planned data interruptions.

The LIGO Scientific Collaboration and the Virgo Collaboration have cataloged eleven confidently detected gravitational-wave events during the first two observing runs of the advanced detector era. All eleven events were consistent with being from well-modeled mergers between compact stellar-mass objects: black holes or neutron stars. The data around the time of each of these events have been made publicly available through the gravitational-wave open science center. The entirety of the gravitational-wave strain data from the first and second observing runs have also now been made publicly available. There is considerable interest among the broad scientific community in understanding the data and methods used in the analyses. In this paper, we provide an overview of the detector noise properties and the data analysis techniques used to detect gravitational-wave signals and infer the source properties. We describe some of the checks that are performed to validate the analyses and results from the observations of gravitational-wave events. We also address concerns that have been raised about various properties of LIGO–Virgo detector noise and the correctness of our analyses as applied to the resulting data.

We propose a multi-mode bar consisting of mass elements of decreasing size for the implementation of a gravitational version of the photo-electric effect through the stimulated absorption of up to kHz gravitons from a binary neutron star merger and post-merger. We find that the multi-mode detector has normal modes that retain the coupling strength to the gravitational wave of the largest mass-element, while only having an effective mass comparable to the mass of the smallest element. This allows the normal modes to have graviton absorption rates due to the tonne-scale largest mass, while the single graviton absorption process in the normal mode could be resolved through energy measurements of a mass-element in-principle smaller than pico-gram scale. We argue the feasibility of directly counting gravito-phonons in the bar through energy measurements of the end mass. This improves the transduction of the single-graviton signal, enhancing the feasibility of detecting single gravitons.

Spurious solar-wind effects are a potential noise source in future Laser Interferometer Space Antenna (LISA) measurements. One noise coupling mechanism is constrained by estimating solar-wind effects on acceleration noise in LISA Pathfinder (LPF). While LISA is designed for drag-free differential measurement, predicting the realistic impact both bounds the operational environment and assesses whether LISA could provide serendipitous space-weather observations. Data from NASA's Advanced Composition Explorer (ACE), situated at the L1 Lagrange point, serves as a reliable source of solar-wind data. The data sets are compared over the 114 d time period from 1 March 2016 to 23 June 2016. This period gives the longest readily-available open data set, without interference from other commissioning activities. To evaluate space weather effects, the data from both satellites are formatted, gap-filled/interpolated, and fast-Fourier transformed for amplitude spectral density and coherence comparisons. Solar wind effects are not seen in a coherence plot between LPF and ACE; modest coherence in the planned LISA observational frequency band can be attributed to chance. This result indicates that measurable correlation due to solar-wind acceleration noise over 3 month timescales will be a negligible noise source. LISA is unlikely to inform solar wind measurements routinely. Another source of noise from the Sun, solar radiation pressure, is estimated to impart greater acceleration noise, but has yet to be analyzed.

We analyze existence and properties of solutions of two-dimensional general relativistic initial data sets with a negative cosmological constant, both on spacelike and characteristic surfaces. A new family of such vacuum spacelike data parameterised by poles at the conformal boundary at infinity is constructed. We review the notions of global Hamiltonian charges, emphasizing the difficulties arising in this dimension, both in a spacelike and characteristic setting. One or two, depending upon the topology, lower bounds for energy in terms of angular momentum, linear momentum, and center of mass are established.

We obtain semiclassical gravity solutions in the Poincaré fundamental domain of 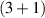$(3+1)$-dimensional Anti-de Sitter spacetime, PAdS₄, with a (massive or massless) Klein–Gordon field (with possibly non-trivial curvature coupling) with Dirichlet or Neumann boundary. Some results are explicitly and graphically presented for special values of the mass and curvature coupling (e.g. minimal or conformal coupling). In order to achieve this, we study in some generality how to perform the Hadamard renormalisation procedure for non-linear observables in maximally symmetric spacetimes in arbitrary dimensions, with emphasis on the stress-energy tensor. We show that, in this maximally symmetric setting, the Hadamard bi-distribution is invariant under the isometries of the spacetime, and can be seen as a 'single-argument' distribution depending only on the geodesic distance, which significantly simplifies the Hadamard recursion relations and renormalisation computations.

Loop quantum gravity (LQG) methods are applied to a symmetry-reduced model with homogeneity in two dimensions, derived from a Gowdy model. The conditions for the propagation of unidirectional plane gravitational waves at exactly the speed of light are set up in the form of two null Killing equations in terms of Ashtekar variables and imposed as operators on the quantum states of the system. Owing to symmetry reduction the gauge group of the system reduces formally from SU(2) to U(1). Under the assumption of equal spacing of the holonomy eigenvalues, the solutions are not normalizable in the sense of the usual inner product on U(1). Taking over the inner product from the genuine gauge group SU(2) of LQG renders the obtained states normalizable, nevertheless fluctuations of geometrical quantities remain divergent. In consequence, the solutions of the (non-commuting) Killing conditions have to be renormalized. Two kinds of renormalization are presented. The combination of the occurrence of non-commuting Killing operators and the necessity of renormalization indicates fluctuations of the propagation speed, i. e. dispersion of gravitational waves. Finally the same methods are applied to the Hamiltonian constraint with the same result concerning normalizability. After renormalization the constraint is not exactly satisfied any more, which suggests the presence of some kind of interacting matter.

We prove that some of the static Myers/Korotkin–Nicolai (MKN) vacuum 3 + 1 static black holes cannot be put into stationary rotation. Namely, they cannot be deformed into axisymmetric stationary vacuum black holes with non-zero angular momentum. We also prove that this occurs in particular for those MKN solutions for which the distance along the axis between the two poles of the horizon is sufficiently small compared to the square root of its area. The MKN solutions, sometimes called periodic Schwarzschild, are physically regular, have no struts or singularite, but are asymptotically Kasner. The static rigidity presented here appears to be the first in the literature of General Relativity.

We compute the leading order corrections to the expected value of the squared field amplitude of a massless real scalar quantum field due to curvature in a localized region of spacetime. We use Riemann normal coordinates to define localized field operators in a curved spacetime that are analogous to their flat space counterparts, and the Hadamard condition to find the leading order curvature corrections to the field correlations. We then apply our results to particle detector models, quantifying the effect of spacetime curvature in localized field probes.

Group field theory is a background-independent approach to quantum gravity whose starting point is the definition of a quantum field theory on an auxiliary group manifold (not interpreted as spacetime, but rather as the finite-dimensional configuration space of a single 'atom' of geometry). Group field theory models can be seen as an extension of matrix and tensor models by additional data, and are traditionally defined through a functional integral whose perturbative expansion generates a sum over discrete geometries. More recently, some efforts have been directed towards formulations of group field theory based on a Hilbert space and operators, in particular in applications to cosmology. This is an attempt to review some of these formulations and their main ideas, to disentangle these constructions as much as possible from applications and phenomenology, and to put them into a wider context of quantum gravity research.

The unification of quantum mechanics and general relativity has long been elusive. Only recently have empirical predictions of various possible theories of quantum gravity been put to test, where a clear signal of quantum properties of gravity is still missing. The dawn of multi-messenger high-energy astrophysics has been tremendously beneficial, as it allows us to study particles with much higher energies and travelling much longer distances than possible in terrestrial experiments, but more progress is needed on several fronts. A thorough appraisal of current strategies and experimental frameworks, regarding quantum gravity phenomenology, is provided here. Our aim is twofold: a description of tentative multimessenger explorations, plus a focus on future detection experiments. As the outlook of the network of researchers that formed through the COST Action CA18108 'Quantum gravity phenomenology in the multi-messenger approach (QG-MM)', in this work we give an overview of the desiderata that future theoretical frameworks, observational facilities, and data-sharing policies should satisfy in order to advance the cause of quantum gravity phenomenology.

Rotating and oscillating neutron stars can give rise to long-lived Continuous Gravitational Waves (CGWs). Despite many years of searching, the detection of such a CGW signal remains elusive. In this article we describe the main astrophysical uncertainties regarding such emission, and their relation to the behaviour of matter at extremely high density. We describe the main challenges in searching for CGWs, and the prospects of detecting them using third-generation gravitational wave detectors. We end by describing some pressing issues in the field, whose resolution would help turn the detection and exploitation of CGWs into reality.

We review the status of mathematical research on the dynamical properties of relativistic fluids in cosmological spacetimes–both, in the presence of gravitational backreaction as well as the evolution on fixed cosmological backgrounds. We focus in particular on the phenomenon of fluid stabilization, which describes the taming effect of spacetime expansion on the fluid. While fluids are in general known to form shocks from regular initial data, spacetime expansion has been found to suppress this behaviour. During the last decade, various rigorous results on this problem have been put forward. We review these results, the mathematical methods involved and provide an outlook on open questions.

Gravitational memory effects and the BMS freedoms exhibited at future null infinity have recently been resolved and utilized in numerical relativity simulations. With this, gravitational wave models and our understanding of the fundamental nature of general relativity have been vastly improved. In this paper, we review the history and intuition behind memory effects and BMS symmetries, how they manifest in gravitational waves, and how controlling the infinite number of BMS freedoms of numerical relativity simulations can crucially improve the waveform models that are used by gravitational wave detectors. We reiterate the fact that, with memory effects and BMS symmetries, not only can these next-generation numerical waveforms be used to observe never-before-seen physics, but they can also be used to test GR and learn new astrophysical information about our Universe.

In the present work, we investigate the Bose-Einstein condensates (BECs) on electrically charged Noncommutative inspired (NCi) black holes (BHs). The NC parameter represents a quantum correction that modifies spacetime geometry by introducing a minimal length scale. This impacts the black hole's effective gravitational field and, consequently, the dynamics of nearby scalar fields. The BEC is represented by a massive scalar field governed by the Klein-Gordon equation with a self-interaction term, assuming the scalar field is uncharged and devoid of self-gravitation and the mass parameter of the scalar field to be sufficiently small that may allow conditions to be a candidate for dark-matter clouds. 
We start our studies by analyzing the properties of the event horizon and the mass profiles of NCi BH inside it.
The BH charge and the NC parameters, $Q/M-\Theta/M^2$, space are also studied. Then, we analyze the effective potential of a test scalar field in both radial and tortoise coordinates for the different values of the BH charge, the NC, and the scalar field mass parameters. Finally, we study the density function in the Thomas-Fermi approximation, in which the condensate is located in a spherical shell. The parameters $Q$ and $\Theta$ can slightly modify the condensed density distribution.

This paper explores the phenomenon of particle creation associated with cosmic strings in de Sitter spacetime, a model that represents the universe's exponential expansion. We examine how the presence of cosmic strings in a de Sitter background affects particle production, focusing on the roles of string tension and angular deficits. Utilizing the Klein-Gordon equation adapted to curved spacetime with cosmic string defects, we derive solutions expressed through hypergeometric functions to describe particle states. Our findings highlight how string properties influence particle creation rates and energy distributions. By analyzing both point-like and linear potentials near the string, we determine exact solutions, investigate asymptotic behaviors, and calculate particle creation probabilities using Bogoliubov transformations.

The impact of space weather on space mission performance is a subject of extensive study by both scientists and space agencies. In this work, we discuss solar activity, the characterization of the interplanetary medium and the fluxes of solar and galactic particles after 2035, when LISA, the first interferometer for low-frequency gravitational wave detection in space, is expected to be launched. According to the lessons learned with LISA Pathfinder, the precursor mission of LISA, we focus on interplanetary phenomena that have been demonstrated to affect mission performance. The results of the study presented here can be considered in any investigation concerning the internal charging of the LISA spacecraft.

The regularization of the scalar constraint and the Fermion coupling problem indicate that it is necessary to consider some kind of gauge fixing methods to deal with the simplicity constraint in all dimensional SO(D + 1) loop quantum gravity. The coherent state with well-behaved peakedness property is an essential ingredient to carry out the gauge fixing method. To provide the basic tool for constructing such kind of coherent state, we generalize the twisted geometry parametrization of the SO(D + 1) holonomy-flux phase space of (1 + D)-dimensional loop quantum gravity from the edge simplicity constraint surface to the full SO(D + 1) holonomy-flux phase space. The symplectic structure on the twisted geometric parameter space and the Poisson structure in terms of the twisted geometric variables are analyzed. Besides, we discuss the relation between the two twisted geometry parametrizations constructed respectively on the edge simplicity constraint surface and the full SO(D + 1) holonomy-flux phase space. Our result shows that these two type of parametrizations are equivalent to each other by carrying out the gauge reduction with respect to the edge simplicity constraint.

Some time ago, Seiberg and Witten solved for moduli spaces of vacua parameterized by scalar vacuum expectation values in N = 2 gauge theories. More recently, new vacua associated to soft theorems and asymptotic symmetries have been found. This paper takes some first steps towards a complete picture of the infrared geometry of N = 2 gauge theory incorporating both of these infrared structures.

We analyze existence and properties of solutions of two-dimensional general relativistic initial data sets with a negative cosmological constant, both on spacelike and characteristic surfaces. A new family of such vacuum spacelike data parameterised by poles at the conformal boundary at infinity is constructed. We review the notions of global Hamiltonian charges, emphasizing the difficulties arising in this dimension, both in a spacelike and characteristic setting. One or two, depending upon the topology, lower bounds for energy in terms of angular momentum, linear momentum, and center of mass are established.

We obtain semiclassical gravity solutions in the Poincaré fundamental domain of 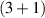$(3+1)$-dimensional Anti-de Sitter spacetime, PAdS₄, with a (massive or massless) Klein–Gordon field (with possibly non-trivial curvature coupling) with Dirichlet or Neumann boundary. Some results are explicitly and graphically presented for special values of the mass and curvature coupling (e.g. minimal or conformal coupling). In order to achieve this, we study in some generality how to perform the Hadamard renormalisation procedure for non-linear observables in maximally symmetric spacetimes in arbitrary dimensions, with emphasis on the stress-energy tensor. We show that, in this maximally symmetric setting, the Hadamard bi-distribution is invariant under the isometries of the spacetime, and can be seen as a 'single-argument' distribution depending only on the geodesic distance, which significantly simplifies the Hadamard recursion relations and renormalisation computations.

We prove that some of the static Myers/Korotkin–Nicolai (MKN) vacuum 3 + 1 static black holes cannot be put into stationary rotation. Namely, they cannot be deformed into axisymmetric stationary vacuum black holes with non-zero angular momentum. We also prove that this occurs in particular for those MKN solutions for which the distance along the axis between the two poles of the horizon is sufficiently small compared to the square root of its area. The MKN solutions, sometimes called periodic Schwarzschild, are physically regular, have no struts or singularite, but are asymptotically Kasner. The static rigidity presented here appears to be the first in the literature of General Relativity.

We compute the leading order corrections to the expected value of the squared field amplitude of a massless real scalar quantum field due to curvature in a localized region of spacetime. We use Riemann normal coordinates to define localized field operators in a curved spacetime that are analogous to their flat space counterparts, and the Hadamard condition to find the leading order curvature corrections to the field correlations. We then apply our results to particle detector models, quantifying the effect of spacetime curvature in localized field probes.

Group field theory is a background-independent approach to quantum gravity whose starting point is the definition of a quantum field theory on an auxiliary group manifold (not interpreted as spacetime, but rather as the finite-dimensional configuration space of a single 'atom' of geometry). Group field theory models can be seen as an extension of matrix and tensor models by additional data, and are traditionally defined through a functional integral whose perturbative expansion generates a sum over discrete geometries. More recently, some efforts have been directed towards formulations of group field theory based on a Hilbert space and operators, in particular in applications to cosmology. This is an attempt to review some of these formulations and their main ideas, to disentangle these constructions as much as possible from applications and phenomenology, and to put them into a wider context of quantum gravity research.

A Wick rotation in the lapse (not in time) is introduced that interpolates between Riemannian and Lorentzian metrics on real
 manifolds admitting a codimension-one foliation. The definition refers to a fiducial foliation but covariance under foliation
 changing diffeomorphisms can be rendered explicit in a reformulation as a rank one perturbation. Applied to scalar field
 theories a Lorentzian signature action develops a positive imaginary part thereby identifying the underlying complex metric
 as `admissible'. This admissibility is ensured in non-fiducial foliations in technically distinct ways also for the variation
 with respect to the metric and for the Hessian. The Hessian of the Wick rotated action is a complex combination of a 
generalized Laplacian and a d'Alembertian, which is shown to have spectrum contained in a wedge of the upper complex 
half plane. Specialized to near Minkowski space the induced propagator differs from the one with the Feynman $i\epsilon$ 
prescription and on Friedmann-Lema\^{i}tre backgrounds the difference to a Wick rotation in time is illustrated.

In their seminal 1992 paper, Bañados, Teitelboim and Zanelli (BTZ) proposed a simple charged generalization of what is now known as the spinning BTZ black hole, the proposal being that a rotating metric can be supported by a `static vector' potential.
While with such an ansatz the Einstein equations are satisfied, and the corresponding energy-momentum tensor is divergence-less, the Maxwell equations do not (due to the special degenerate form of the corresponding field strength) hold. More recently, Deshpande and Lunin have proposed a generalized `Einstein--Maxwell' system which yields analytic rotating black holes in all odd dimensions. In this paper, we show that the original charged BTZ solution can be re-interpreted as a solution of the Deshpande--Lunin theory. Moreover, as we shall explicitly illustrate on an example of regularized conformal electrodynamics, similar construction also works for any non-linear electrodynamics in 3-dimensions.

We provide a characterisation of the Kerr spacetime close to future null infinity using the asymptotic characteristic initial value problem in a conformally compactified spacetime. Stewart's gauge is used to set up the past-oriented characteristic initial value problem. By a theorem of M. Mars characterising the Kerr spacetime, we provide conditions for the existence of an asymptotically timelike Killing vector on the development of the initial data by demanding that the spacetime is endowed with a Killing spinor. The conditions on the characteristic initial data ensuring the existence of a Killing spinor are, in turn, analysed. Finally, we write the conditions on the initial data in terms of the free data in the characteristic initial value problem. As a result, we characterise the Kerr spacetime using only a section of future null infinity and its intersection with an outgoing null hypersurface.

We construct an effective cosmological spin-foam model for a (2+1) dimensional spatially flat universe, discretized on a cubical lattice, containing both space- and time-like regions. Our starting point is the recently proposed coherent state spin-foam model for (2+1) Lorentzian quantum gravity. The full amplitude is assumed to factorize into single vertex amplitudes with boundary data corresponding to Lorentzian 3-frusta. A stationary phase approximation is performed at each vertex individually, where the inverse square root of the Hessian determinant serves as a measure for the effective path integral. Additionally, a massive scalar field is coupled to the geometry, and we show that its mass renders the partition function convergent. For a single 3-frustum with time-like struts, we compute the expectation value of the bulk strut length and show that it generically agrees with the classical solutions and that it is a discontinuous function of the scalar field mass. Allowing the struts to be space-like introduces causality violations, which drive the expectation values away from the classical solutions due to the lack of an exponential suppression of these configurations. This is a direct consequence of the semi-classical amplitude only containing the real part of deficit angles, in contrast with the Lorentzian Regge action used in effective spin-foams. We give an outlook on how to evaluate the partition function on an extended discretization including a bulk spatial slice. This serves as a foundation for future investigations of physically interesting scenarios such as a quantum bounce or the viability of massive scalar field clocks. Our results demonstrate that the effective path integral in the causally regular sector serves as a viable quantum cosmology model, but that the agreement of expectation values with classical solutions is tightly bound to the path integral measure.

We present a covariant description of non-vacuum static spherically symmetric spacetimes in $f(R)$ gravity applying the (1+1+2) covariant formalism. The propagation equations are then used to derive a covariant and dimensionless form of the Tolman-Oppenheimer-Volkoff (TOV) equations. We then give a solution strategy to these equations and obtain some new exact solutions for the particular case f(R)=R+α R², which have the correct thermodynamic properties for standard matter.