Reply to the Comment by Andrés G. Jirón Vicente et al.

In response to the Comment [1], we show here that the energy eigenvalues obtained in Cases A, B in the original paper [2] are correct while it is not so for the one obtained in Case C which is correctly presented here. We disagree with the energy eigenvalues presented in all cases in the Comment. It is worth mentioning here that there is a typo in the normalized radial wave function in the original paper [2]) which we correctly present here.

The Klein-Gordon equation under Lorentz symmetry violation defined by a fixed vector field 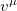$v^{\mu}$ is described by (replacing coupling constant $g \to \beta$ here)

$$\begin{eqnarray} &&\Bigg[\frac{1}{\sqrt{-g}}\,\partial_{\mu}\,(\sqrt{-g}\,g^{\mu\nu}\,\partial_{\nu})-\beta\,(v^{\mu}\,\partial_{\mu})^2-M^2\Bigg]\,\Psi=0, \end{eqnarray} \tag{ 1 }$$

The KG-oscillator can be studied by replacing the operator 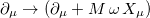$\partial_{\mu} \to (\partial_{\mu}+M\,\omega\,X_{\mu})$ [2,3] in the above equation. Therefore, the KG-oscillator under Lorentz symmetry violation is described by the following wave equation:

$$\begin{eqnarray} & \Bigg[\frac{1}{\sqrt{-g}}\,(\partial_{\mu}+M\,\omega\,X_{\mu})\,(\sqrt{-g}\,g^{\mu\nu})(\partial_{\nu}-M\,\omega\,X_{\nu})\nonumber\\ & -\beta\,(v^{\mu}\,\partial_{\mu})^2-M^2\Bigg]\,\Psi=0, \end{eqnarray} \tag{ 2 }$$

where we have defined in polar coordinates 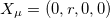$X_{\mu}=(0, r, 0, 0)$ with g the determinant of the metric tensor and 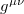$g^{\mu\nu}$ the inverse metric tensor. We imposed the condition that the fixed vector field satisfies 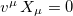$v^{\mu}\,X_{\mu}=0$ [2]. The Minkowski metric in polar coordinates is of the form 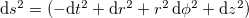$\mathrm{d}s^2=(-\mathrm{d}t^2+\mathrm{d}r^2+r^2\,\mathrm{d}\phi^2+\mathrm{d}z^2)$ with

$$\begin{eqnarray} &&g_{\mu\nu}=\mathrm{diag}(-1, 1, r^2, 1),\quad g^{\mu\nu}=\mathrm{diag}\left(-1, 1, \frac{1}{r^2}, 1\right)\!\!. \end{eqnarray} \tag{ 3 }$$

Therefore, the KG-oscillator equation in the background (3) becomes

$$\begin{eqnarray} &&\Bigg[\!-\!\!\frac{\partial^2}{\partial t^2}\!+\!\nabla^2\!-\!M^2\,\omega^2\,r^2\!-\!2M\omega \!-\!\beta(v^{\mu}\partial_{\mu})^2\!-\!M^2 \Bigg]\!\Psi\!=\!0,\quad \end{eqnarray} \tag{ 4 }$$

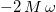where $\nabla^2=[\frac{1}{r}\,\frac{\partial}{\partial r}\,(r\,\frac{\partial}{\partial r})+\frac{1}{r^2}\,\frac{\partial^2}{\partial \phi^2}+\frac{\partial^2}{\partial z^2}]$. The above equation is exactly similar to eq. (3) in the original paper [2] (please see γ in eq. (5) in ref. [3], where a term $-2\,M\,\omega$ appears for the KG-oscillator equation). In ref. [4], we have shown how this term 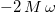$-2\,M\,\omega$ will appear in the KG-oscillator.

In Case A, the vector field is chosen by 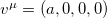$v^{\mu}=(a, 0, 0, 0)$ and solves the radial equation using the NU-method. The energy eigenvalue expression is given by (see eq. (10) in ref. [2])

$$\begin{eqnarray} &&E_{n,l}\!=\!\pm\,\sqrt{\frac{1}{(1+a^2\,\beta)}\,\Bigg[M^2+k^2+4\,M\,\omega\,\Big(n+\frac{|l|}{2}+1\Big)\Bigg]}\!. \end{eqnarray} \tag{ 5 }$$

Now, we will check the correctness of this energy eigenvalue expression. Let 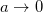$a \to 0$, that is, without Lorentz symmetry violation effect. Therefore, the energy eigenvalue (5) reduces to

$$\begin{eqnarray} &&E_{n,l}=\pm\,\sqrt{M^2+k^2+4\,M\,\omega\,\Big(n+\frac{|l|}{2}+1\Big)} \end{eqnarray} \tag{ 6 }$$

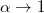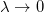which is similar to the energy eigenvalue obtained in ref. [3] (see eq. (12) in ref. [3] by substituting $\alpha \to 1$ and $\lambda \to 0$ there), whereas the eigenvalue presented in the Comment never reduces to that result (6) for $a \to 0$. There are no doubts that the energy eigenvalues obtained in ref. [3] are incorrect. Hence, the energy eigenvalue in Case A in the original paper [2] is correct while we disagree with the claim made in the Comment [1].

The normalized radial wave function is given by

$$\begin{eqnarray} &&\psi_{n,l}(x)=\sqrt{2\,M\,\omega}\,\sqrt{\frac{n!}{(n+l)!}}\,x^{\frac{|l|}{2}}\,e^{-\frac{x}{2}}\,L^{(|l|)}_{n}(x). \end{eqnarray} \tag{ 7 }$$

In the original expression in ref. [2], the multiplicative term 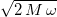$\sqrt{2\,M\,\omega}$ was missing, basically a typo there.

In terms of r, where 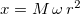$x=M\,\omega\,r^2$, one can write

$$\begin{eqnarray} \psi_{n,l} (r)&= \sqrt{\frac{2\,(n)!}{(n+l)!}}\,(M\,\omega)^{\frac{|l|+1}{2}}\nonumber\\* & \quad \times\,r^{|l|}e^{-\frac{1}{2}\,M\,\omega\,r^2}\,L^{(|l|)}_{n}(M\,\omega\,r^2), \end{eqnarray} \tag{ 8 }$$

which satisfies the normalization condition 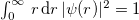$\int^{\infty}_{0}\,r\,\mathrm{d}r\,|\psi(r)|^2=1$.

The total wave function will be

$$\begin{eqnarray} \Psi_{n,l} (t, r, \phi, z)\!&= \!\sqrt{2}\,\sqrt{\frac{n!}{(n+l)!}}\,e^{i\,(-E_{n,l}\,t+l\,\phi+k\,z)}\nonumber\\ & \quad\times\,(M\,\omega)^{\frac{|l|+1}{2}}\,r^{|l|}\,e^{-\frac{1}{2}\,M\,\omega\,r^2}\,L^{(|l|)}_{n}(M\,\omega\,r^2), \end{eqnarray} \tag{ 9 }$$

where E_{n, l} is given in eq. (5). This total wave function is influenced by the Lorentz symmetry violation defined by the fixed vector field 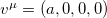$v^{\mu}=(a, 0, 0, 0)$ but not the radial function.

Similarly, one can show that the energy eigenvalue presented in Case B in the original paper [2] is correct. The normalized radial wave function is the same given by (7), whereas the total wave function will be

$$\begin{eqnarray} \Psi_{n,l} (t, r, \phi, z)\!&= \!\sqrt{\frac{2\,(n)!}{(n+l)!}}\,e^{i\,(-E_{n,l}\,t+l\,\phi+k\,z)}\nonumber\\ & \quad\times\,(M\,\omega)^{\frac{|l|+1}{2}}\,r^{|l|}\,e^{-\frac{1}{2}\,M\,\omega\,r^2}\,L^{(|l|)}_{n}(M\,\omega\,r^2), \end{eqnarray} \tag{ 10 }$$

where E_{n, l} is given in Case B in ref. [2]. The total wave function is influenced by the Lorentz symmetry violation defined by the fixed vector field 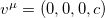$v^{\mu}=(0, 0, 0, c)$.

For the chosen vector field 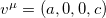$v^{\mu}=(a, 0, 0, c)$ in Case C in the original paper [2], the correct energy eigenvalue expression is given by

$$\begin{eqnarray} & E_{n,l}=\frac{a\,c\,k\,\beta}{(1+a^2\,\beta)}\pm \frac{1}{(1+a^2\,\beta)}\,\Bigg\{a^2\,c^2\,k^2\,\beta^2\nonumber\\ & +\,(1+a^2\,\beta)\,\Big[M^2+k^2\,(1-c^2\,\beta)\nonumber\\ & +\,4\,M\,\omega\,\Big(n+\frac{|l|}{2}+1\Big)\Big]\Bigg\}^{1/2}. \end{eqnarray} \tag{ 11 }$$

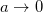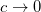One can easily show that for $a \to 0$ and $c \to 0$, this eigenvalue (11) reduces to the result (6). We therefore disagree with the energy expression presented in the Comment [1].

The normalized radial wave function will be the same given by (7), whereas the total wave function will be

$$\begin{eqnarray} \Psi_{n,l} (t, r, \phi, z)&= \sqrt{\frac{2\,(n)!}{(n+l)!}}\,e^{i\,(-E_{n,l}\,t+l\,\phi+k\,z)}\nonumber\\ & \quad\times\,(M\,\omega)^{\frac{|l|+1}{2}}\,r^{|l|}\,e^{-\frac{1}{2}\,M\,\omega\,r^2}\,L^{(|l|)}_{n}(M\,\omega\,r^2), \end{eqnarray} \tag{ 12 }$$

where E_{n, l} is given by eq. (11). The total wave function is influenced by the Lorentz symmetry violation defined by the fixed vector field 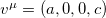$v^{\mu}=(a, 0, 0, c)$.

We have shown that the energy eigenvalue expression presented in Cases A, B in the original paper [2] agree with the known result obtained earlier without the Lorentz symmetry violation effects. While for Case C in the original paper [2], the correct energy expression is given by eq. (11) which is different from the result presented in the Comment. In addition, the normalized radial functions in all cases are the same as that given by eq. (7) but the total wave functions Ψ given by eqs. (9), (10) and (12) are different due to the influence of the Lorentz symmetry violation defined by the fixed vector field 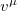$v^{\mu}$ but not the radial function. It is better to mention here that the energy eigenvalues obtained in ref. [3] are absolutely correct, which confirms our results, too. Thus, we disagree with the results presented in the Comment [1].

The author sincerely thanks the anonymous referee for valuable suggestions.