Abstract
Recently, we have shown the existence of a finite energy one-half monopole. In this paper, we would like to introduce electric charge into the one-half monopole configuration, thus creating a one-half dyon. This one-half dyon possesses finite energy, a magnetic dipole moment and angular momentum. Hence it is able to rotate in the presence of an external magnetic field. Similarly to single pole dyons and MAP dyons, this one-half dyon possesses a critical (maximum) electric charge, total energy and magnetic dipole moment when the Higgs self-coupling constant is non-vanishing and the electric charge parameter approaches one. This one-half dyon solution does not satisfy the first order Bogomol'nyi equations and is a non-BPS solution in the limit of a vanishing Higgs self-coupling constant.
Export citation and abstract BibTeX RIS
1. Introduction
In Maxwell theory, the monopole carries a Dirac string. This monopole, which is the Dirac monopole [1], possesses infinite energy and obeys the quantization condition  , where M is the magnetic charge, e is the U(1) gauge field coupling constant and N is an integer. However when the gauge field is extended from the Abelian U(1) to the non-Abelian SU(2) Yang–Mills field in the presence of a Higgs field in the adjoint representation, the Dirac string disappears and the monopole's energy becomes finite [2]. This monopole, which is the 't Hooft–Polyakov monopole, possesses a magnetic charge of one and is radially symmetrical. In the SU(2) Yang–Mills–Higgs (YMH) field theory in 3 + 1 dimensions, the quantization condition becomes
, where M is the magnetic charge, e is the U(1) gauge field coupling constant and N is an integer. However when the gauge field is extended from the Abelian U(1) to the non-Abelian SU(2) Yang–Mills field in the presence of a Higgs field in the adjoint representation, the Dirac string disappears and the monopole's energy becomes finite [2]. This monopole, which is the 't Hooft–Polyakov monopole, possesses a magnetic charge of one and is radially symmetrical. In the SU(2) Yang–Mills–Higgs (YMH) field theory in 3 + 1 dimensions, the quantization condition becomes  , where g is the SU(2) gauge field coupling constant.
, where g is the SU(2) gauge field coupling constant.
The SU(2) YMH field theory possesses various configurations of monopole solutions which are invariant under a U(1) subgroup of the local SU(2) gauge group. The invariance under a U(1) subgroup is important as there must be symmetry breaking of the theory into the Abelian subgroup which will give rise to Maxwell's electromagnetic field theory as was mentioned by 't Hooft in his paper [2]. Since the work of Dirac [1] and 't Hooft and Polyakov [2] hundreds of papers on magnetic monopoles have been written. Exact monopole and multimonopole solutions were shown to exist in the Bogomol'nyi–Prasad–Sommerfield (BPS) limit [3] when the Higgs potential vanishes. Numerical BPS monopole solutions with no rotational symmetry were discussed in [4]. Other numerical finite energy solutions with axial symmetry, such as the monopole–antimonopole pair (MAP), monopole–antimonopole chain (MAC) and vortex ring solutions [5] with both vanishing and non-vanishing Higgs field self-coupling constants, were also shown to exist in this theory. Recently, numerical generalized Jacobi elliptic single monopole solutions and numerical generalized Jacobi elliptic MAP and single vortex ring solutions were also found in this theory [6].
Most of the monopole solutions discussed in the literature are of integer topological magnetic charge. However there are some papers with discussions on one-half monopoles. These include the work of Harikumar et al [7]. They demonstrated the existence of generic smooth YM potentials of one-half monopoles. However no exact or numerical solutions have been given. Exact one-half monopole axially symmetric solutions and one-half monopole mirror symmetric solutions with Dirac-like strings were discussed in [8]. However these exact solutions possess infinite total energy.
Recently, we have found a finite energy one-half monopole solution [9]. The 't Hooft magnetic fields of these solutions at spatial infinity correspond to the magnetic field of a positive one-half magnetic monopole located at the origin, r = 0, and a semi-infinite Dirac string located on one half of the z-axis which carries magnetic flux  going into the center of the sphere at infinity. Hence the net magnetic charge of the configuration is zero. The non-Abelian solutions possess gauge potentials that are singular along one half of the z-axis, elsewhere they are regular. The total energy of this one-half magnetic monopole solution is found to increase with λ.
going into the center of the sphere at infinity. Hence the net magnetic charge of the configuration is zero. The non-Abelian solutions possess gauge potentials that are singular along one half of the z-axis, elsewhere they are regular. The total energy of this one-half magnetic monopole solution is found to increase with λ.
The word 'dyon' was coined by Schwinger in 1969 [10] for a particle that possesses both magnetic and electric charges. A dyon with a fixed magnetic charge can possess varying electric charges [11] at the classical level. The dyon solutions of Julia and Zee [12] are time-independent solutions that possess non-vanishing kinetic energy. The Julia–Zee solutions are non-self-dual even in the BPS limit when the electric charge is non-vanishing. Outside the BPS limit when λ is non-vanishing, the Julia–Zee solutions are of course non selfdual. The exact dyon solutions found by Prasad and Sommerfield [12] are actually Julia and Zee dyon solutions in the BPS limit. These solutions are stable as they are the absolute minima of the energy [13].
By applying the standard procedure of introducing electric charge into the theory as shown by Julia and Zee [12] all the monopole solutions of the SU(2) YMH theory can acquire an electric charge to become a dyon. Single pole dyons with axial symmetry were constructed by Hartmann et al [14] when the electric charge parameter η is varied from zero to one. These axial dyons are actually generalized 't Hooft–Polyakov monopoles that possess magnetic charges n = 1, 2, 3 and non-vanishing electric charges when the parameter η is nonzero. It was found in [14] that when the strength of the Higgs potential λ is non-vanishing, the total electric charge and total energy of the system approach finite critical values when the parameter η approaches one. However when λ = 0, the total electric charge and total energy approach infinity when the parameter η approaches one.
Recently in [15], we also found that when λ is non-vanishing, the electric charge, total energy and magnetic dipole moment of the zero topological charge sector of the MAP and vortex ring solutions approach finite critical values when the electric charge parameter η approaches one. Similarly when λ = 0, the electric charge, total energy and magnetic dipole moment approach infinity when η approaches one. The MAP dyons were also investigated in [16] by varying λ for a fixed value of η.
Since the one-half monopole solution of [9] is a new finite energy solution with properties that differ from the integer topological magnetic charge monopoles, we would like to further study its properties and behavior when electric charges are introduced into the theory. This can be done by using the standard procedure of Julia and Zee [12] for the magnetic ansatz as was done in [14, 15]. We calculate numerically for the dimensionless electric charge Q, the dimensionless total energy E, the dimensionless angular momentum Jz about the z-axis of symmetry, as well as the dimensionless magnetic dipole moment μm of the one-half dyon solution when the electric charge parameter η is varied from zero to one. Since this one-half dyon possesses a finite magnetic dipole moment and angular momentum, it is able to rotate in the presence of an external magnetic field. Similarly to the single pole dyons [14] and the MAP dyons [15], this one-half dyon possesses critical (maximum) electric charge, total energy and magnetic dipole moment for the non-vanishing Higgs self-coupling constant, λ, when the electric charge parameter, η, approaches one. When λ vanishes, these quantities approach infinity when η approaches one. We also calculate for the total energy E, the total electric charge Q and the magnetic dipole moment μm of the one-half dyon solution for fixed values of η and for λ from zero to 12. In general, the total electric charge and magnetic dipole moment decrease with increasing λ, however the total energy decreases until λ ≈ 1 and increases with increasing λ.
We briefly review the SU(2) YMH field theory in the next section. In section 3, we discuss the construction of the one-half dyon solution. The magnetic ansatz used in obtaining the one-half dyon solution and some of its basic properties are discussed in this section. The numerical results of our calculations of the one-half dyon solution are presented and discussed in section 4. We end with some comments in section 5.
2. The SU(2) YMH theory
The SU(2) YMH Lagrangian in 3+1 dimensions with a non-vanishing Higgs potential is given by
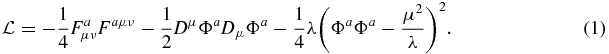
Here the Higgs field mass is μ and the strength of the Higgs potential is λ, both of which are constants. The vacuum expectation value of the Higgs field is  . The Lagrangian (1) is gauge invariant under the set of independent local SU(2) transformations at each space-time point. The covariant derivative of the Higgs field and the gauge field strength tensor are given respectively by
. The Lagrangian (1) is gauge invariant under the set of independent local SU(2) transformations at each space-time point. The covariant derivative of the Higgs field and the gauge field strength tensor are given respectively by

where g is the gauge field coupling constant. The metric used is gμν = ( − + + +). The SU(2) internal group indices a, b, c = 1, 2, 3 and the space-time indices are μ, ν, α = 0, 1, 2, and 3 in Minkowski space. The equations of motion that follow from the Lagrangian (1) are
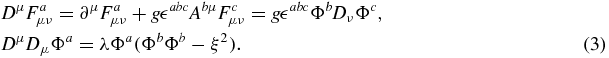
In the limit of vanishing μ and λ, the Higgs potential vanishes and self-dual solutions can be obtained by solving the first order partial differential Bogomol'nyi equation,
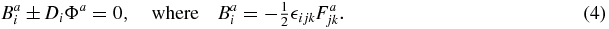
The electromagnetic field tensor proposed by 't Hooft [2] upon symmetry breaking is
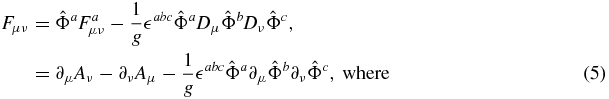
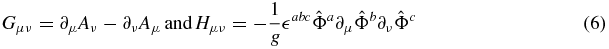
are the gauge part and the Higgs part of the electromagnetic field respectively. Here  , the Higgs unit vector,
, the Higgs unit vector,  and the Higgs field magnitude
and the Higgs field magnitude  . Hence the decomposed magnetic field is
. Hence the decomposed magnetic field is

where BGi and BHi are the gauge part and Higgs part of the magnetic field respectively. The net magnetic charge of the system is
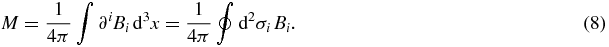
The topological magnetic current, [17]
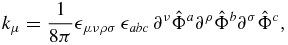
is also the topological current density of the system. Hence the corresponding conserved topological magnetic charge is
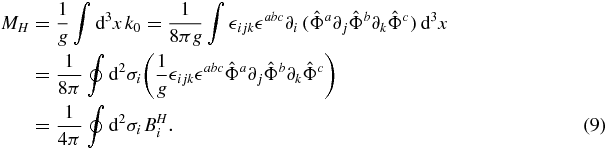
The magnetic charge MH is the total magnetic charge of the system if and only if the gauge field is non singular [18]. If the gauge field is singular and carries Dirac string monopoles MG, then the total magnetic charge of the system is
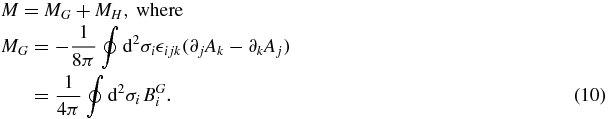
3. The construction of the solution
3.1. The magnetic ansatz
The time independent gauge fields and Higgs field of the magnetic ansatz that leads to the one-half dyon solutions are given respectively by [14, 15]
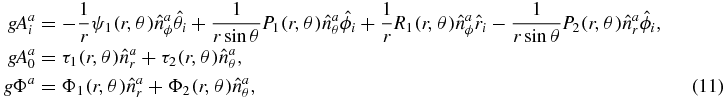
where P1(r, θ) = sin θψ2(r, θ) and P2(r, θ) = sin θR2(r, θ). We choose to perform our numerical analysis with the functions P1(r, θ) and P2(r, θ) to avoid the line singularity of R2(r, θ). The spatial spherical coordinate orthonormal unit vectors are
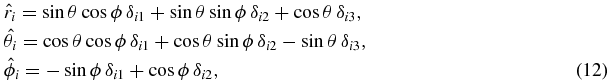
and the isospin coordinate orthonormal unit vectors are
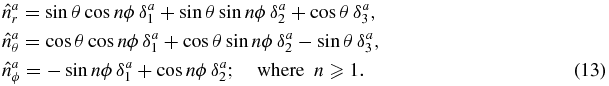
The ϕ-winding number n is a natural number. In our work here on the one-half dyon we take n = 1. The magnetic ansatz (11) is form invariant under the gauge transformation
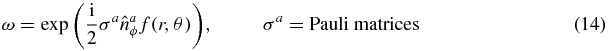
and the transformed gauge potential and Higgs field take the form
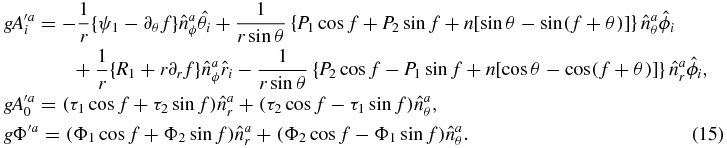
3.2. The Higgs field
The general Higgs fields in the spherical and the rectangular coordinate systems are
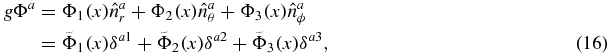
respectively, where
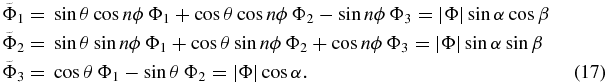
The axially symmetric Higgs unit vector in the rectangular coordinate system is
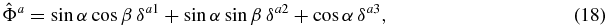
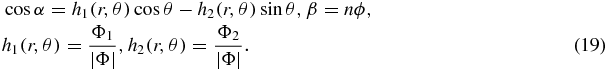
From equations (18) and (19), the Higgs field (11) and the gauge transformed Higgs field (15) can be written in terms of α as
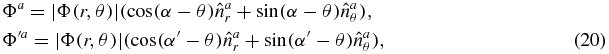
where α' = α − f.
3.3. The magnetic field and charge
By using the definition of cos α (19), the Higgs part of the 't Hooft magnetic field (7) can be reduced to

The gauge part of the magnetic field (7) can be written in a similar form
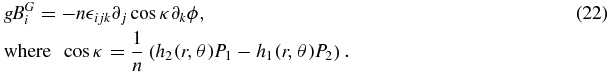
Hence the 't Hooft magnetic field which is the sum of the Higgs part (21) and the gauge part (22) is given by
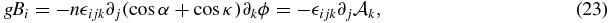
where  is the 't Hooft gauge potential. The magnetic field lines of the configuration can be plotted by drawing the contour lines of (cos α + cos κ) = constant on the vertical plane ϕ = 0. The orientation of the magnetic field can also be plotted by using the vector plot of the magnetic field unit vector
is the 't Hooft gauge potential. The magnetic field lines of the configuration can be plotted by drawing the contour lines of (cos α + cos κ) = constant on the vertical plane ϕ = 0. The orientation of the magnetic field can also be plotted by using the vector plot of the magnetic field unit vector
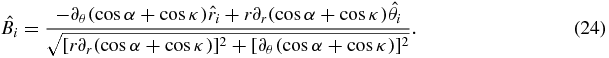
At spatial infinity in the Higgs vacuum, all the non-Abelian components of the gauge potential vanish and the non-Abelian electromagnetic field tends to
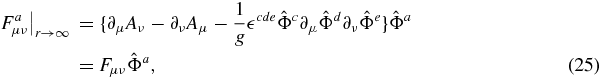
where Fμν is just the 't Hooft electromagnetic field. However according to Coleman [19], there is no unique way of representing the Abelian electromagnetic field in the region of the monopole outside the Higgs vacuum at finite values of r. One proposal was given by 't Hooft as in equation (5). The 't Hooft magnetic field has the special property that the magnetic charge density vanishes, that is ∂iBi = 0, when  . However when |Φ| = 0,
. However when |Φ| = 0,  and the magnetic charges are located at these points. Hence with 't Hooft's definition of the electromagnetic field, the magnetic charges are discrete and reside at the point zeros of the Higgs field. Since this definition gives a discrete magnetic charge at a particular point, there is no magnetic charge distribution over space. Another proposal for the Abelian electromagnetic field was given by Bogomol'nyi [2] and Faddeev [20]. In this definition which is less singular, the magnetic and electric fields are given respectively by
and the magnetic charges are located at these points. Hence with 't Hooft's definition of the electromagnetic field, the magnetic charges are discrete and reside at the point zeros of the Higgs field. Since this definition gives a discrete magnetic charge at a particular point, there is no magnetic charge distribution over space. Another proposal for the Abelian electromagnetic field was given by Bogomol'nyi [2] and Faddeev [20]. In this definition which is less singular, the magnetic and electric fields are given respectively by

where ξ is the vacuum expectation value of the Higgs field. Hence instead of becoming singular,  and
and  vanish when the Higgs field vanishes. With this definition of the electromagnetic field (26) there will be a magnetic charge density distribution contributed by the non-Abelian components of the gauge field in the finite r region. Since the magnetic charge density of the one-half dyon solution is singular and yet integrable, we therefore define the weighted magnetic charge density to be
vanish when the Higgs field vanishes. With this definition of the electromagnetic field (26) there will be a magnetic charge density distribution contributed by the non-Abelian components of the gauge field in the finite r region. Since the magnetic charge density of the one-half dyon solution is singular and yet integrable, we therefore define the weighted magnetic charge density to be  . A 3D surface plot of the weighted magnetic charge density,
. A 3D surface plot of the weighted magnetic charge density,  , distribution will be useful in determining the sign of the magnetic charge of the solutions. In the Higgs vacuum at spatial infinity, both definitions of the electromagnetic field (5) and (26) become similar.
, distribution will be useful in determining the sign of the magnetic charge of the solutions. In the Higgs vacuum at spatial infinity, both definitions of the electromagnetic field (5) and (26) become similar.
The topological magnetic charge (9), or the magnetic charge carried by the Higgs field can be evaluated by the expression

Similarly to the one-half monopole configuration [9], the magnetic charge of the one-half dyon is the positive half and the Dirac string carries the other negative half of the magnetic charge. Hence the net magnetic charge of the configuration is zero, M = MH + MG = 0.
3.4. The electric field and charge
Using definition (26) by Bogomol'nyi [2] and Faddeev [20], we similarly define the weighted electric charge density to be  . The weighted electric charge density distribution
. The weighted electric charge density distribution  can be calculated and plotted numerically, where
can be calculated and plotted numerically, where  is the electric field. The weighted electric charge density,
is the electric field. The weighted electric charge density,  , of the dyon solution is solely positive throughout space when η is positive.
, of the dyon solution is solely positive throughout space when η is positive.
At spatial infinity in the Higgs vacuum,
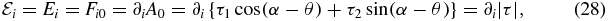
where  , since the time component of the gauge field, Aa0, is assumed parallel to the Higgs field, Φa, in isospin space. Hence both definitions for the electromagnetic field strength will give the same total electric charge, Q(λ, η), with
, since the time component of the gauge field, Aa0, is assumed parallel to the Higgs field, Φa, in isospin space. Hence both definitions for the electromagnetic field strength will give the same total electric charge, Q(λ, η), with  less singular than Ei at finite values of r. Unlike the magnetic field, the electric field varies proportionally with the constant 0 ⩽ η < 1. The electric field can therefore be switched off by setting η = 0.
less singular than Ei at finite values of r. Unlike the magnetic field, the electric field varies proportionally with the constant 0 ⩽ η < 1. The electric field can therefore be switched off by setting η = 0.
From Gauss' law, the total electric charge of the dyon in units of 4πξ is given by,  . Since we assume that Aa0 is parallel to the Higgs field at large r, then [14],
. Since we assume that Aa0 is parallel to the Higgs field at large r, then [14],  , can be calculated numerically. An alternative way to find Q is to assume that
, can be calculated numerically. An alternative way to find Q is to assume that  , where a is a constant, at large r. Then Q = ηξa1 can be obtained by plotting r(|τ| − ηξ) and reading off the value of ηξa1 at large r. In our case, we choose to evaluate Q by numerically evaluating the volume integration
, where a is a constant, at large r. Then Q = ηξa1 can be obtained by plotting r(|τ| − ηξ) and reading off the value of ηξa1 at large r. In our case, we choose to evaluate Q by numerically evaluating the volume integration

3.5. The magnetic dipole moment and the angular momentum
From Maxwell electromagnetic theory, the 't Hooft gauge potential,  , of equation (23) at large r tends to
, of equation (23) at large r tends to
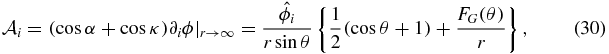
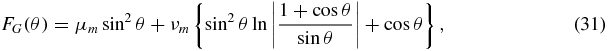
where μm is the dimensionless magnetic dipole moment of the one-half monopole. From the numerical solution, FG(θ) can be calculated numerically using the expression
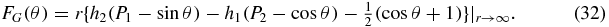
Plotting the graphs of FG(θ) versus angle θ, we find that FG(θ) = μmsin 2θ, and μm is non-vanishing for all values of λ and 0 ⩽ η < 1. The constant, νm, is however zero. The value of μm is read from the graphs of FG(θ) versus angle θ at  . The magnetic dipole moment, μm, was obtained for various values of 0 ⩽ η < 1 and 0 < λ ⩽ 12.
. The magnetic dipole moment, μm, was obtained for various values of 0 ⩽ η < 1 and 0 < λ ⩽ 12.
The energy momentum tensor of the YMH theory is given by
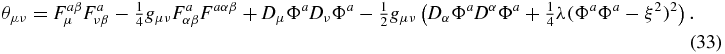
For the magnetic ansatz (11), the Poynting vector of the system can be reduced to [15], [16]
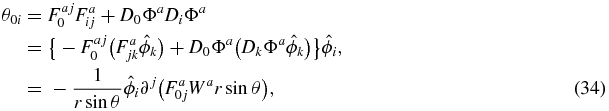
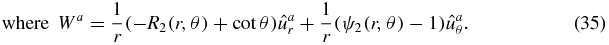
The angular momentum density along the z-axis is given by
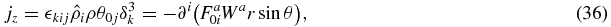
where  and
and  . Hence the total angular momentum in units of 4πξ is
. Hence the total angular momentum in units of 4πξ is
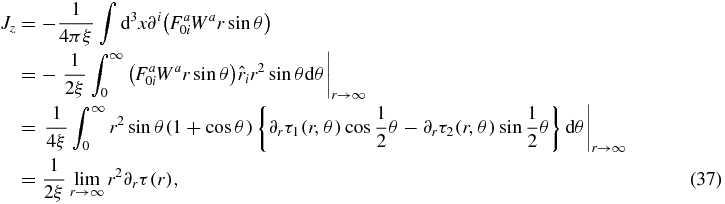
if we assume that the time component of the gauge field, Aa0, is parallel to the Higgs field, Φa, at spatial infinity. Then  and
and  as r → ∞. Hence from equation (37),
as r → ∞. Hence from equation (37),  when ξ = 1 and the one-half dyon solutions possess kinetic energy of rotation.
when ξ = 1 and the one-half dyon solutions possess kinetic energy of rotation.
3.6. The energy
In the electrically charged BPS limit when the Higgs potential vanishes, the energy which is a minimum is given by [14]

where MH is the 'topological magnetic charge' and Q as given by equation (29) is the total electric charge of the system when the vacuum expectation value of the Higgs field, ξ, is nonzero. Since the one-half dyon solution is not a BPS solution even in the limit of vanishing λ, its energy must be greater than that given by equation (38). Its dimensionless value is given by
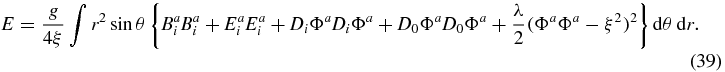
Since the one-half dyon solution possesses an integrable singular energy density, we define the weighted energy density to be
3.7. Numerical calculations
We solve for the numerical one-half dyon solution by substituting the ansatz (11) into the equations of motion (3). The 15 equations of motion are then reduced to eight coupled nonlinear second order partial differential equations. These eight equations are solved asymptotically first at small and then at large distances.
The numerical one-half dyon solution is constructed by modifying the exact type A1 one-half magnetic monopole solution [9] to include the electric charge parameter, 0 ⩽ η ⩽ 1,
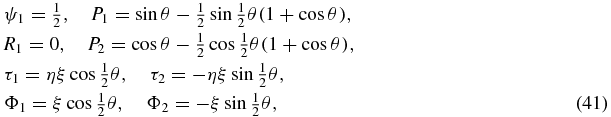
and using it as an asymptotic solution at large distances (r → ∞). In this asymptotic region, the time component of the gauge field and the Higgs field are parallel in the isospin space, that is Φ1∝τ1 and Φ2∝τ2.
Near the origin, r = 0, we have the common trivial vacuum solution. The asymptotic solution and boundary conditions at small distances that will give rise to a finite energy one-half dyon solution are
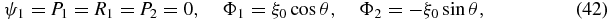
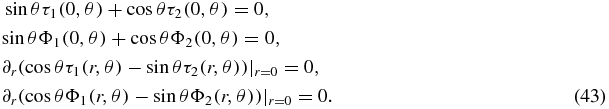
The boundary conditions imposed along the positive z-axis for the profile functions (11) of the type A1 one-half dyon solution are
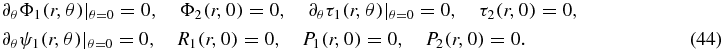
Along the negative z-axis, the boundary conditions imposed are
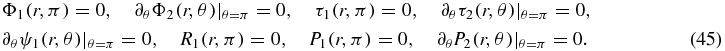
In our numerical calculation we choose to set the expectation value ξ and the gauge coupling constant g to one. The numerical one-half dyon solution connecting the exact asymptotic solution (41) at large distances to the trivial vacuum solution (42) at small distances and subjected to the boundary conditions (43)–(45) for the type A1 solution together with the gauge fixing condition [5]

are solved using the Maple and MATLAB software [19]. The eight reduced second order partial differential equations of motion are then transformed into a system of nonlinear equations using the finite difference approximation. The numerical calculations are performed with the system of nonlinear equations discretized on a non-equidistant grid of size 90 × 80 covering the integration regions  and 0 ⩽ θ ⩽ π. Here
and 0 ⩽ θ ⩽ π. Here  is the compactified finite interval coordinate. The partial derivatives with respect to the radial coordinate are then replaced accordingly by
is the compactified finite interval coordinate. The partial derivatives with respect to the radial coordinate are then replaced accordingly by  and
and  . We then used Maple to find the Jacobian sparsity pattern for the system of nonlinear equations. This information is given to MATLAB to run the numerical computation. The system of nonlinear equations are then solved numerically using the trust-region-reflective algorithm by providing the solver with a good initial guess.
. We then used Maple to find the Jacobian sparsity pattern for the system of nonlinear equations. This information is given to MATLAB to run the numerical computation. The system of nonlinear equations are then solved numerically using the trust-region-reflective algorithm by providing the solver with a good initial guess.
The second order equations of motion (3) are solved when the ϕ-winding number n = 1, the electric charge parameter 0 ⩽ η < 1 and with the Higgs potential such that the Higgs self-coupling constant 0 ⩽ λ ⩽ 12. In our calculations, there are two kinds of error, the first inherited from the finite difference approximation of the functions, which is of the order of 10−4. The second comes from the linearization of the nonlinear equations for MATLAB to solve numerically; the remainder or approximation error is of the order of 10−6. Hence the overall error estimate is 10−4.
4. Numerical results
The profile functions, ψ1, R1, P1, P2, τ1, τ2, Φ1 and Φ2 obtained for the numerical one-half dyon solution are all regular functions of r and θ. However R2 is singular along the negative z-axis. This is similar to the type A1 one-half monopole [9] as when the electric charge parameter, η, is set to zero the dyon becomes the type A1 one-half monopole.
We calculate and plot for the Higgs field modulus, |Φ|, the weighted energy density,  , the weighted magnetic charge density,
, the weighted magnetic charge density,  , and the weighted electric charge density,
, and the weighted electric charge density,  , versus the x–z plane at y = 0 numerically. The 3D surface plot of these physical quantities and their respective contour plot are shown in figures 1(a) and (b) and figures 2(a) and (b), respectively when λ = 1 and η = 0.9. In general, the shape of the dyon remains the same as that of the one-half monopole [9] while its size increases as η increases from zero to one. The situation is similar to that of an elongated balloon with the negative z-axis as its axis of symmetry been blown from its opening at the origin, r = 0.
, versus the x–z plane at y = 0 numerically. The 3D surface plot of these physical quantities and their respective contour plot are shown in figures 1(a) and (b) and figures 2(a) and (b), respectively when λ = 1 and η = 0.9. In general, the shape of the dyon remains the same as that of the one-half monopole [9] while its size increases as η increases from zero to one. The situation is similar to that of an elongated balloon with the negative z-axis as its axis of symmetry been blown from its opening at the origin, r = 0.
Figure 1. (a) The 3D surface and contour plots of the Higgs field modulus, |Φ|, along the x–z plane at y = 0. (b) The 3D surface and contour plots of the weighted energy density,  , along the x–z plane at y = 0. Here λ = 1 and η = 0.9.
, along the x–z plane at y = 0. Here λ = 1 and η = 0.9.
Download figure:
Standard imageFigure 2. (a) The 3D surface and contour plots of the weighted magnetic charge density,  , along the x–z plane at y = 0. (b) The 3D surface and contour plots of the weighted electric charge density,
, along the x–z plane at y = 0. (b) The 3D surface and contour plots of the weighted electric charge density,  , along the x–z plane at y = 0. Here λ = 1 and η = 0.9.
, along the x–z plane at y = 0. Here λ = 1 and η = 0.9.
Download figure:
Standard imageWe also notice that as the electric charge parameter η increases from zero to one, the zeros of the Higgs modulus along the negative z-axis increase and the inverted cone becomes more stretched along the negative z-axis. The peak value of the weighted energy density,  , shown in figure 1(b) is 1.221 (1.087 when η = 0) and it occurs along the z-axis at z = −3.647 (z = −2.235 when η=0) [9]. From figures 2(a) and (b), we can conclude that the one-half dyon carries both positive magnetic and electric charge densities that are concentrated along the negative z-axis near the origin.
, shown in figure 1(b) is 1.221 (1.087 when η = 0) and it occurs along the z-axis at z = −3.647 (z = −2.235 when η=0) [9]. From figures 2(a) and (b), we can conclude that the one-half dyon carries both positive magnetic and electric charge densities that are concentrated along the negative z-axis near the origin.
The vector field plots of the Higgs field, Φa, the electric field unit vector,  , and the magnetic field unit vector,
, and the magnetic field unit vector,  , along the x–z plane at y = 0 when λ = 1 and η = 0.9 are shown in figures 3(a), (b) and (d) with the location of the one-half dyon marked by *. The contour plot of the magnetic field lines is shown in figure 3(c). The plot is almost similar to the case with no electric charge (η = 0) [9] except that magnetic flux concentration along the negative z-axis close to the origin is longer when η = 0.9 or when Q = 0.47.
, along the x–z plane at y = 0 when λ = 1 and η = 0.9 are shown in figures 3(a), (b) and (d) with the location of the one-half dyon marked by *. The contour plot of the magnetic field lines is shown in figure 3(c). The plot is almost similar to the case with no electric charge (η = 0) [9] except that magnetic flux concentration along the negative z-axis close to the origin is longer when η = 0.9 or when Q = 0.47.
Figure 3. (a) The vector plot of the Higgs field vector, Φa, along the x–z plane at y = 0. (b) The vector plot of the electric field unit vector,  , along the x–z plane at y = 0. (c) The contour plot of the magnetic field lines along the x–z plane at y = 0. (d) The vector plot of the magnetic field unit vector,
, along the x–z plane at y = 0. (c) The contour plot of the magnetic field lines along the x–z plane at y = 0. (d) The vector plot of the magnetic field unit vector,  , along the x–z plane at y = 0. Here the * indicates the location of the one-half dyon, λ = 1 and η = 0.9.
, along the x–z plane at y = 0. Here the * indicates the location of the one-half dyon, λ = 1 and η = 0.9.
Download figure:
Standard imageWe obtained numerically the total electric charge, Q, the magnetic dipole moment, μm, and the total energy, E, when λ = 0, 1, and 0 ⩽ η < 1 and these values are tabulated in table 1. The graphs of Q, μm and E versus η are then plotted as shown in figure 4. From the graphs of figures 4(a)– (c), we can conclude that the values of Q, μm and E increase to infinity as η → 1 when λ vanishes. However when λ is non-vanishing, these values approach critical values as η → 1. The critical values when λ = 1 are Qc = 0.59, μmc = 1.56 and Ec = 0.96. Similar conclusions are also reported in [14, 15].
Figure 4. (a) Plots of the total electric charge, Q, versus η. (b) Plots of the magnetic dipole moment, μm, versus η. (c) Plots of the total energy, E, versus η. (d) Plots of the magnetic dipole moment, μm, versus Q. (e) Plots of the total energy, E, versus Q. (f) Plots of the magnetic dipole moment, μm, versus E. Here λ = 0 and 1, 0 ⩽ η < 1.
Download figure:
Standard imageTable 1. The electric charge Q, the dimensionless magnetic dipole moment μm and the dimensionless total energy E of the one-half dyon for different values of η at λ = 0 and λ = 1.0.
| λ = 1.0 | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| η | 0 | 0.05 | 0.1 | 0.15 | 0.2 | 0.40 | 0.80 | 0.90 | 0.94 | 0.99 | 1.00 |
| Q | 0 | 0.02 | 0.04 | 0.06 | 0.08 | 0.16 | 0.38 | 0.47 | 0.52 | 0.57 | 0.59 |
| μm | 1.06 | 1.06 | 1.06 | 1.07 | 1.07 | 1.11 | 1.32 | 1.42 | 1.47 | 1.54 | 1.56 |
| E | 0.59 | 0.59 | 0.60 | 0.60 | 0.60 | 0.63 | 0.77 | 0.84 | 0.88 | 0.94 | 0.95 |
| λ = 0 | |||||||||||
| η | 0 | 0.05 | 0.1 | 0.15 | 0.2 | 0.40 | 0.80 | 0.90 | 0.94 | 0.99 | 1.00 |
| Q | 0 | 0.02 | 0.05 | 0.07 | 0.09 | 0.20 | 0.61 | 0.95 | 1.26 | 3.38 | – |
| μm | 1.32 | 1.32 | 1.33 | 1.34 | 1.35 | 1.44 | 2.20 | 3.02 | 3.85 | 9.56 | – |
| E | 0.51 | 0.51 | 0.51 | 0.51 | 0.52 | 0.55 | 0.82 | 1.12 | 1.43 | 3.58 | – |
From the graphs of μm versus Q (figure 4(d)) and μm versus E (figure 4(f)), we find that for a fixed value of Q or E, the magnetic dipole moment of the one-half dyon at λ = 0 is always higher than that of μm when λ = 1. However the reverse is true for the case of E versus Q as shown in figure 4(e). The total energy of the one-half dyon, E, when λ = 1 is always higher than that of E when λ = 0 for a particular value of total electric charge, Q. We also note that the type A1 one-half dyon possesses higher total energy than the energy of the BPS limit one-half dyon, EBPS which is plotted using equation (39).
The values of E, Q and μm for various values of η = 0.5, 0.9, 1 are tabulated in table 2 when 0 ⩽ λ ⩽ 12 and plotted versus  as shown in figures 5(a)–(c) respectively. From these graphs, we are able to conclude that E(η2) > E(η1), Q(η2) > Q(η1) and μm(η2) > μm(η1), if η2 > η1. We also note that for small values of η ⩽ 0.5 the total energy of the one-half dyon increases as λ increases. However for higher values of η ⩾ 0.5, the total energy, E, decreases then increases as λ increases. However the total electric charge and magnetic dipole moment decrease as λ increases.
as shown in figures 5(a)–(c) respectively. From these graphs, we are able to conclude that E(η2) > E(η1), Q(η2) > Q(η1) and μm(η2) > μm(η1), if η2 > η1. We also note that for small values of η ⩽ 0.5 the total energy of the one-half dyon increases as λ increases. However for higher values of η ⩾ 0.5, the total energy, E, decreases then increases as λ increases. However the total electric charge and magnetic dipole moment decrease as λ increases.
Figure 5. (a) Plot of the total energy, E, versus  when η = 0, 0.5, 0.75, 0.9, 1. (b) Plot of the total electric charge, Q, versus
when η = 0, 0.5, 0.75, 0.9, 1. (b) Plot of the total electric charge, Q, versus  when η = 0.5, 0.9, 1. (c) Plot of the magnetic dipole moment, μm, versus
when η = 0.5, 0.9, 1. (c) Plot of the magnetic dipole moment, μm, versus  when η = 0.5, 0.9, 1. (d) Plot of the total magnetic charge, M, the magnetic charge carried by the Higgs field, MH, and the magnetic charge carried by the gauge field, MG, versus the compactified coordinate,
when η = 0.5, 0.9, 1. (d) Plot of the total magnetic charge, M, the magnetic charge carried by the Higgs field, MH, and the magnetic charge carried by the gauge field, MG, versus the compactified coordinate,  , when λ = 1 and η = 0, 1.
, when λ = 1 and η = 0, 1.
Download figure:
Standard imageTable 2. The electric charge Q, the dimensionless magnetic dipole moment μm and the dimensionless total energy E of the one-half dyon for different values of λ at η = 0.5, 0.9 and 1.0.
| η = 1.0 | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| λ | 0 | 0.04 | 0.09 | 0.20 | 0.40 | 0.80 | 1.00 | 2.00 | 4.00 | 8.00 | 12.00 |
| Q | ∞ | 0.85 | 0.77 | 0.70 | 0.65 | 0.60 | 0.59 | 0.55 | 0.52 | 0.49 | 0.48 |
| μm | ∞ | 2.37 | 2.12 | 1.91 | 1.75 | 1.60 | 1.56 | 1.43 | 1.32 | 1.22 | 1.16 |
| E | ∞ | 1.11 | 1.05 | 1.01 | 0.98 | 0.96 | 0.96 | 0.95 | 0.94 | 0.94 | 0.95 |
| η = 0.9 | |||||||||||
| λ | 0 | 0.04 | 0.09 | 0.20 | 0.40 | 0.80 | 1.00 | 2.00 | 4.00 | 8.00 | 12.00 |
| Q | 0.95 | 0.63 | 0.58 | 0.54 | 0.51 | 0.48 | 0.47 | 0.44 | 0.42 | 0.40 | 0.39 |
| μm | 3.02 | 1.96 | 1.82 | 1.67 | 1.56 | 1.46 | 1.42 | 1.32 | 1.23 | 1.15 | 1.10 |
| E | 1.12 | 0.89 | 0.87 | 0.85 | 0.85 | 0.84 | 0.84 | 0.84 | 0.85 | 0.86 | 0.87 |
| η = 0.5 | |||||||||||
| λ | 0 | 0.04 | 0.09 | 0.20 | 0.40 | 0.80 | 1.00 | 2.00 | 4.00 | 8.00 | 12.00 |
| Q | 0.27 | 0.24 | 0.23 | 0.22 | 0.22 | 0.21 | 0.21 | 0.20 | 0.19 | 0.19 | 0.18 |
| μm | 1.52 | 1.36 | 1.31 | 1.26 | 1.21 | 1.16 | 1.15 | 1.10 | 1.04 | 0.99 | 0.96 |
| E | 0.56 | 0.50 | 0.61 | 0.62 | 0.63 | 0.64 | 0.66 | 0.68 | 0.70 | 0.71 | 0.72 |
In figure 5(d), the total magnetic charge M = MH + MG, the magnetic charge carried by the Higgs field, MH, (9) and the magnetic charge carried by the gauge field, MG, (10) are plotted versus the compactified coordinate,  , when λ = 1 and η = 0, 1. We notice that for the electrically neutral, η = 0, one-half dyon (or the one-half monopole [9]), the magnetic charges M and MH approach
, when λ = 1 and η = 0, 1. We notice that for the electrically neutral, η = 0, one-half dyon (or the one-half monopole [9]), the magnetic charges M and MH approach  and MG → 0 faster than the fully electrically charged η = 1 one-half dyon as
and MG → 0 faster than the fully electrically charged η = 1 one-half dyon as  .
.
5. Comments
Van Der Bij and Radu [16] conjectured the absence of rotating regular solitons with a net magnetic charge and also argued that there are no axially symmetric rotating monopole solutions for a YMH theory in a flat space-time background. In the single pole dyon solutions of reference [14], which are electrically charged 't Hooft–Polyakov monopoles when n = 1, the configurations do not possess a magnetic dipole moment or angular momentum. However in the one-MAP dyons of reference [15], the configurations possess both a magnetic dipole moment and angular momentum about the axis of symmetry which is the z-axis. The angular momentum of the one-MAP dyons about the z-axis is Jz = nQ. Hence the one-MAP dyons which possess zero net magnetic charge are able to rotate but the single pole dyons that possess a non-vanishing net magnetic charge cannot rotate in the presence of an external magnetic field.
Similarly to the MAP dyon, the one-half dyon possesses both a magnetic dipole moment and angular momentum. The angular momentum of the one-half dyon about the z-axis is  as n = 1. Hence although the one-half dyon is a single pole structure and possesses a net magnetic charge, it is able to rotate in the presence of an external magnetic field. This is possible because the gauge potentials of the one-half dyon possess a semi-infinite string singularity.
as n = 1. Hence although the one-half dyon is a single pole structure and possesses a net magnetic charge, it is able to rotate in the presence of an external magnetic field. This is possible because the gauge potentials of the one-half dyon possess a semi-infinite string singularity.
Similarly to the dyon solutions of [14, 15], the total electric charge, the magnetic dipole moment and the total energy of the one-half dyon increases indefinitely as the electric charge parameter η → 1 when λ vanishes. However when λ is non-vanishing, Q, μm and E increase to maximum critical values as given in tables 1 and 2.
Our numerical calculations for the total energy of the one-half dyon when λ = 0 show that its total energy, E, is slightly higher than the theoretical minimum BPS limit energy, EBPS, by 2% when Q = 0 and four percent when Q = 0.6. Hence similarly to the one-half monopole solution of [9], these one-half dyon solutions are not the BPS limit solutions even though λ vanishes.
Acknowledgments
The authors would like to thank Universiti Sains Malaysia for the RU research grant (account number: 1001/PFIZIK/811180).





