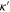Abstract
Color codes are topological stabilizer codes with unusual transversality properties. Here I show that their group of transversal gates is optimal and only depends on the spatial dimension, not the local geometry. I also introduce a generalized, subsystem version of color codes. In 3D they allow the transversal implementation of a universal set of gates by gauge fixing, while error-dectecting measurements involve only four or six qubits.
Export citation and abstract BibTeX RIS

Content from this work may be used under the terms of the Creative Commons Attribution 3.0 licence. Any further distribution of this work must maintain attribution to the author(s) and the title of the work, journal citation and DOI.
1. Introduction
In fault-tolerant quantum computation [1], quantum information is protected from noise by encoding it in somewhat non-local degrees of freedom, thus distributing it among many smaller subsystems, typically qubits. This makes sense under the physically relevant assumption that interactions with the environment have a local nature. The implementation of gates, consequently, must be also as local as possible to preserve the structure of noise. This is naturally achieved with transversal gates, i.e. unitary operators that transform encoded states by acting separately on suitable subsystems, in the simplest case independently on each qubit. Unfortunately, no code admits a universal transversal set of gates, i.e. such that transversal gates can approximate arbitrary gates [2].
Topological quantum error correcting codes [3] introduce a richer notion of locality by considering the spatial location of the physical qubits, which are assumed to be arranged on a lattice. They come in families parametrized with a lattice size, for a fixed spatial dimension. Their defining features are (i) that the measurements needed to recover information about errors only involve a few neighbouring qubits and (ii) that no encoded information can be recovered without access to a number of physical qubits comparable to the system size. Rather than sticking to transversal operations, for topological codes it is natural to consider instead local operations quantum circuits of fixed depth with geometrically local gates [4, 5].
Remarkably, spatial dimension constrains the gates that can be implemented by such local means. This is in particular true for topological stabilizer codes, a popular class of codes for which it is often possible to obtain general results, as exemplified by the classification of 2D codes [6, 7]1
. Along this line, it was shown recently [5] that for D-dimensional topological stabilizer codes all local gates belong to the set 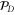 , defined recursively [8] setting
, defined recursively [8] setting 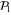 to be the Pauli group
to be the Pauli group 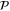 of operators and
of operators and 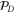 to be the set of unitary gates U with
to be the set of unitary gates U with

This result constraints the gates can be implemented locally, but unfortunately it says nothing about which valid gates can be realized in some topological stabilizer code.
Color codes are a class of topological stabilizer codes with remarkable transversality properties [9]. The original color codes [10] were defined for D = 2, with the aim of making the Clifford group 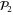 of gates transversal (see [11] for a recent experimental implementation). But color codes can also be defined for any
of gates transversal (see [11] for a recent experimental implementation). But color codes can also be defined for any 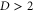 on lattices called D-colexes [12]. If colexes fulfilling certain local conditions can be constructed, families of color codes exist on dimension D that admit the transversal implementation of the gates CNot and
on lattices called D-colexes [12]. If colexes fulfilling certain local conditions can be constructed, families of color codes exist on dimension D that admit the transversal implementation of the gates CNot and 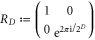 [13, 14]. Since
[13, 14]. Since 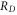 belongs to
belongs to 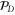 but not to
but not to 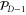 , color codes could saturate the geometrical constraint.
, color codes could saturate the geometrical constraint.
The difficulty is that it is not obvious that D-colexes with the required local conditions can be constructed for any D. A first aim of this work is to show that actually such local conditions are irrelevant the transversality properties of a color code are independent of the local geometry of the colex. This is interesting at a theoretical level because D-colexes can be easily built for any dimension [12] and thus the geometrical constraint on local gates is saturated2 . Notice in this regard that gates are not only local but also transversal. On the practical side, with less constraints on the choice of the lattice of physical qubits more efficient codes are possible. In 2D, for example, there exist families of color codes that for error correction only require measurements involving up to 6 physical qubits each. With the constraints enforced, the number of qubits goes up to 8 [10].
Since no code admits a universal set of transversal gates, we are forced to find alternate routes. A popular approach is the distillation of noisy magic states [15] (for which 2D color codes are well suited since the whole Clifford group is transversal). It has the advantage of its quite general applicability, but the disadvantage that most resources end up being used for distillation, rather than the intended computation. More efficient techniques are thus desirable, and in this regard two recent developments have been the gauge fixing technique [16] and the concatenation of different codes [17].
But tricks to recover universal sets of gates have long been known. In [18] a code is considered such that the CNot and R3 gates are transversal and the initialization and measurement in the computational and Hadamard rotated basis requires only transversal operations and error correction. This suffices to complete the universal gate set with the Hadamard gate, at the price that each Hadamard requires an ancillary encoded qubit. The same technique applies to 3D color codes; indeed, it motivated their introduction. From a practical perspective, however, 3D color codes pose two difficulties. One is the mentioned requirement of ancillas. The other is that the error-detecting measurements can involve each dozens of qubits generally speaking, operations involving more qubits tend to be more unreliable and lengthy.
Both problems are removed in this work by introducing a subsystem form of color codes, gauge color codes. Recall that in a conventional code quantum information is stored in a subspace of the Hilbert space corresponding to the physical qubits. In a subsystem code, instead, this code subspace contains both logical and gauge qubits. The latter are just qubits that we do not care about, and in a topological code they might include local degrees of freedom. This extra degrees of freedom can be put to work at least in two ways. First, error detection measurements are potentially simplified by involving the gauge degrees of freedom [19]. In the case of 3D gauge color codes, this materializes in measurements involving only 4 or 6 physical qubits, just as in 2D. Second, as it was noticed recently [16], it might be possible to perform different transversal gates depending on the state of the gauge qubits. This gauge fixing technique applies to gauge color codes very neatly. In particular for 3D gauge color codes it yields the same universal set of gates described above for conventional 3D color codes but without the need to use ancillary encoded states. An important aspect is that gauge fixing is similar to error correction it only requires local quantum operations supplemented with classical computation.
2. Gates in stabilizer codes
2.1. Stabilizer codes
Stabilizer codes [20, 21] are a main object of study due to their balance of flexibility and simplicity. Given a system of n qubits, a stabilizer subgroup  , with
, with  , defines a subspace, or code, of states
, defines a subspace, or code, of states 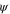 with
with  for every
for every 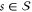 . A subsystem stabilizer code [22] is defined by giving in addition a gauge group
. A subsystem stabilizer code [22] is defined by giving in addition a gauge group  such that
such that  is the center of
is the center of  up to phases. The subspace stabilized by
up to phases. The subspace stabilized by  splits in two subsystems the gauge group generates the full algebra of operators on one of them and acts trivially on the other. Logical qubits (those to be protected) inhabit the later, gauge qubits the former. The elements of
splits in two subsystems the gauge group generates the full algebra of operators on one of them and acts trivially on the other. Logical qubits (those to be protected) inhabit the later, gauge qubits the former. The elements of  , i.e. the group of Pauli operators that commute with the elements of
, i.e. the group of Pauli operators that commute with the elements of  , are called bare logical operators they only act on logical qubits. Elements of
, are called bare logical operators they only act on logical qubits. Elements of 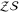 are their dressed counterpart they may act on gauge qubits. Both quotients
are their dressed counterpart they may act on gauge qubits. Both quotients 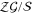 and
and  yield the Pauli group on logical qubits.
yield the Pauli group on logical qubits.
2.2. Transversal gates
Let Xq, Zq denote the Pauli operators X, Z acting on the qubit q, and similarly
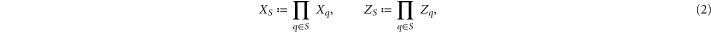
for a set S of qubits. Of interest here are stabilizer codes with (i) a CSS structure [23, 24]  has a generating set
has a generating set 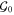 such that each of its elements takes the form XS or ZS for some S, and (ii) a single encoded qubit with bare logical operators XQ, ZQ, where Q is the set of all physical qubits. For such codes the CNot gate is trivially transversal, and also the Hadamard gate if in addition the code is self-dual, i.e.
such that each of its elements takes the form XS or ZS for some S, and (ii) a single encoded qubit with bare logical operators XQ, ZQ, where Q is the set of all physical qubits. For such codes the CNot gate is trivially transversal, and also the Hadamard gate if in addition the code is self-dual, i.e. 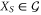 if and only if
if and only if  .
.
More interesting is the gate 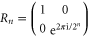 . Let
. Let 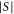 denote the cardinality of a set S. As shown in appendix
denote the cardinality of a set S. As shown in appendix  is transversal if the set Q can be divided into two disjoint sets T and T', i.e.
is transversal if the set Q can be divided into two disjoint sets T and T', i.e.  , such that for every collection of generators
, such that for every collection of generators 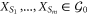 , where
, where 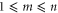 ,
,
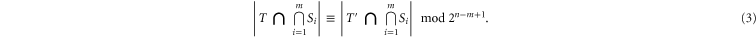
2.3. Gauge fixing
The idea of the gauge fixing technique [16] is that by fixing some of the gauge degrees of freedom to certain values it might be possible to recover transversal gates that would otherwise be forbidden. A code  is a gauge fixed version of
is a gauge fixed version of  if
if 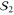 extends
extends 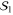 by fixing the values of some operators in
by fixing the values of some operators in  , i.e.
, i.e.
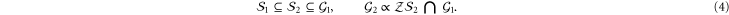
The relations (4) become most transparent by choosing canonical generators  of the Pauli group,
of the Pauli group,  such that
such that
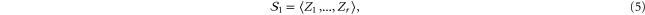
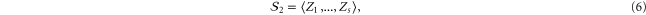
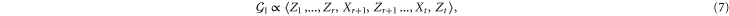
where 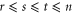 . Computing centralizers is a trivial task, e.g.
. Computing centralizers is a trivial task, e.g.
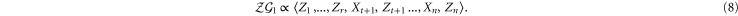
In particular, as canonical generators of the logical Pauli group for the code 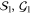 one can take the following representatives of
one can take the following representatives of 

Now, according to (4) we should take
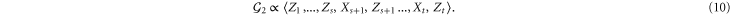
This is indeed a valid choice  is the center of
is the center of 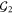 up to phases. Moreover, the operators (9) are clearly also canonical generators of the logical Pauli group for the code
up to phases. Moreover, the operators (9) are clearly also canonical generators of the logical Pauli group for the code  .
.
Since 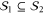 and the two codes share logical operators, encoded states of the second code can be regarded as being encoded states of the first. Conversely, given an encoded state of the first code it is possible to make it into an encoded state of the second code without affecting the logical operators. This amounts to fix the eigenvalues of
and the two codes share logical operators, encoded states of the second code can be regarded as being encoded states of the first. Conversely, given an encoded state of the first code it is possible to make it into an encoded state of the second code without affecting the logical operators. This amounts to fix the eigenvalues of  to +1, which can be done in two steps. First the
to +1, which can be done in two steps. First the  need to be measured, or equivalently any other generators of
need to be measured, or equivalently any other generators of  . Then a product of a subset of the operators
. Then a product of a subset of the operators  is applied, or equivalently any other generators of
is applied, or equivalently any other generators of 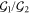 . In particular, Xi is applied if the measurement yields
. In particular, Xi is applied if the measurement yields  . All the operations involved commute with the logical operators (9), and therefore are safe to perform.
. All the operations involved commute with the logical operators (9), and therefore are safe to perform.
An alternative characterization of (4) is possible in terms of logical operators. Suppose that the two codes share a set  of representatives of bare logical operators. Then (4) holds (up to irrelevant phases in
of representatives of bare logical operators. Then (4) holds (up to irrelevant phases in 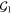 ) if and only if
) if and only if
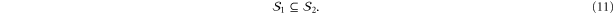
Alternatively, under the same assumption (11) holds (up to a choice of signs in the stabilizers and irrelevant phases in 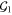 ) if and only if
) if and only if
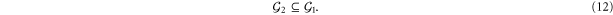
To check the statements (11), (12), notice first that for any code 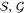 a set
a set 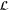 of representatives of bare logical operators satisfies
of representatives of bare logical operators satisfies
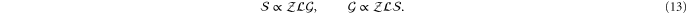
The equivalence of (11) and (12) under the assumption that the two codes share such a set  follows from the basic properties (13). Indeed, (12) implies that
follows from the basic properties (13). Indeed, (12) implies that
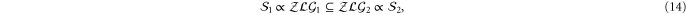
and conversely (11) implies
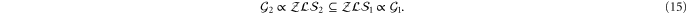
According to the above discussion, if (4) is satisfied there exist such a shared set  and (11) holds. Conversely, if
and (11) holds. Conversely, if 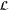 is shared and (11) holds then up to phases
is shared and (11) holds then up to phases
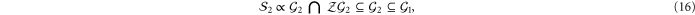
which used (12) and completes the first part of (4), and
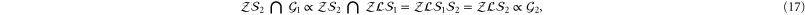
which is the second part of (4).
3. Color codes
3.1. Simplicial lattice
Color codes require lattices called colexes [12], but here the focus is shifted to their dual lattices. For those readers already familiar with colexes, the correspondence is clarified in appendix
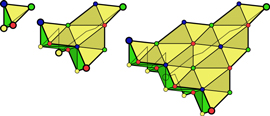
For simplicity we focus on the 2D case. The key to the construction are triangles (2-simplices) with the vertices (0-simplices) labeled with 3 given colors (thus each side or 1-simplex is labeled by 2 colors). We are interested in simplicial lattices (triangulations) with the overall shape of such a colored triangle M. The lattices must have 3-colored 0-simplices such that (i) each 2-simplex is properly colored and (ii) the sides of M have the same coloring as the 1-simplices that lie on them. In figure 1 the first examples of an infinite sequence of such lattices is given: they are obtained by cutting triangles of different sizes out of the same infinite triangular lattice.
Figure 1. The first three instances of an infinite family of lattices for 2D color codes. A qubit is attached to each triangle, thick link and large vertex. Triangles in the set T are shadowed.
Download figure:
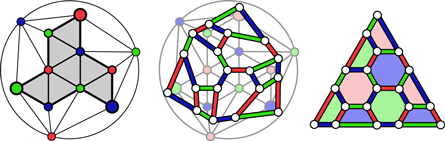
Standard image High-resolution imageFrom every such triangulation one can get a 3-colored triangulation of a sphere just by adding 3 extra 0-simplices as shown in figure 2: there is one new 2-simplex for each 1-simplex on the boundary of M, one for each of the vertices of M, and one formed by the 3 new 0-simplices. It is out of this triangulation of the sphere that a color code can be built, in particular via certain sets  :
:  contains all d-simplices in the new triangulation except those that do not contain any of the original 0-simplices of M. Notice in particular that the elements of
contains all d-simplices in the new triangulation except those that do not contain any of the original 0-simplices of M. Notice in particular that the elements of  correspond one to one to the following elements of M: all 2-simplices, 1-simplices lying on the sides of M, and 0-simplices that are also vertices of M.
correspond one to one to the following elements of M: all 2-simplices, 1-simplices lying on the sides of M, and 0-simplices that are also vertices of M.
Figure 2. Triangulation of a sphere built from a 'triangle' M (shadowed) the hidden half of the sphere is a 2-simplex.
Download figure:
Standard image High-resolution imageThe general D-dimensional case is entirely analogous, with D-simplices instead of triangles and  colors instead of 3. In particular, every d-simplex is labeled by
colors instead of 3. In particular, every d-simplex is labeled by  colors. For a formal exposition see appendix
colors. For a formal exposition see appendix
The 3D case is the most important, so here is a construction where a tetrahedron is carved out of a suitably colored BCC lattice. Vertices are placed at points  or
or  , where
, where 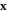 has integer coordinates and
has integer coordinates and 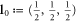 . Red, green, blue and yellow vertices have positions
. Red, green, blue and yellow vertices have positions 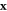 such that
such that 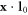 is 0,
is 0,  ,
,  and
and  modulo 1, respectively. Any 3-simplex has vertices of the form
modulo 1, respectively. Any 3-simplex has vertices of the form

where 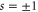 and the triad
and the triad  is a permutation of the triad
is a permutation of the triad 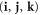 of canonical vectors. Given a positive integer n, retain those simplices with vertices
of canonical vectors. Given a positive integer n, retain those simplices with vertices  such that
such that
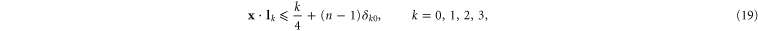
where  ,
,  ,
,  . This gives a tetrahedron: each constraint produces a face with coloring dictated by k, see figure 3.
. This gives a tetrahedron: each constraint produces a face with coloring dictated by k, see figure 3.
Figure 3. The first three instances of an infinite family of 3D color codes. Qubits are attached to all 3-simplices, to 2-simplices covering the external faces, to 1-simplices displayed thicker and to 0-simplices displayed larger. 2-simplices are colored with the complementary to their 3 colors. Those that are darker belong to the set T2.
Download figure:
Standard image High-resolution image3.2. Gauge color codes
Consider sets of d-simplices  from a D-dimensional construction as above,
from a D-dimensional construction as above,  . Attach a physical qubit to each D-simplex in
. Attach a physical qubit to each D-simplex in 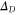 , also denoted Q, and define the groups of operators
, also denoted Q, and define the groups of operators

where 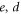 are positive integers,
are positive integers,  ,
,  is the set of D-simplices that contain δ, and
is the set of D-simplices that contain δ, and  denotes a group with generators A. The groups (20) satisfy
denotes a group with generators A. The groups (20) satisfy
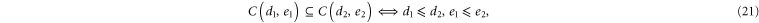
and their centralizer takes the form

where ∝ denotes equality up to global phase [14]. Moreover,  is odd and the group
is odd and the group  has trivial intersection with any of the groups
has trivial intersection with any of the groups  .
.
For positive integers  with
with 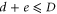 , the (d, e) gauge color code is defined by
, the (d, e) gauge color code is defined by
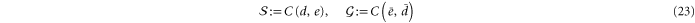
where  . According to the above properties the definition is valid:
. According to the above properties the definition is valid:  is abelian and the center of
is abelian and the center of  . Whatever the values of
. Whatever the values of 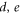 , there is a single encoded qubit with logical Pauli operators
, there is a single encoded qubit with logical Pauli operators  . When
. When 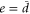 the color code is conventional [14]. Conventional color codes yield all the possible quotient groups appearing in the construction of logical operators. In particular, it follows from the results on conventional color codes of [12] that logical operators are always non-local: those of the form XS are
the color code is conventional [14]. Conventional color codes yield all the possible quotient groups appearing in the construction of logical operators. In particular, it follows from the results on conventional color codes of [12] that logical operators are always non-local: those of the form XS are 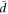 -brane-net like if bare and e-brane-net like if dressed, and those of the form ZS are
-brane-net like if bare and e-brane-net like if dressed, and those of the form ZS are  -brane-net like if bare and d-brane-net like if dressed.
-brane-net like if bare and d-brane-net like if dressed.
Some properties of the above 2D and 3D examples can be extracted from the first instances of each family. For example, in the 2D case the number of qubits is 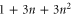 , and in the 3D case it is
, and in the 3D case it is  . Also, in 2D the generators of
. Also, in 2D the generators of 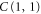 have support on at most 6 qubits, and the same holds for
have support on at most 6 qubits, and the same holds for 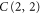 in 3D.
in 3D.
3.3. Transversality
For gauge color codes the CNot gate is transversal. For self-dual codes, d = e, the Hadamard gate is transversal. What about the gate  ? As shown in appendix
? As shown in appendix

In particular, 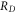 can be implemented by taking
can be implemented by taking 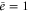 . The result (24) was obtained for
. The result (24) was obtained for  and
and  in [14], but an important limitation there was the lack of a general recipe to construct lattices satisfying (3).
in [14], but an important limitation there was the lack of a general recipe to construct lattices satisfying (3).
As it turns out the set T only depends on the lattice, not the specific (d, e) parameters of the code. It must be such that the operator XT, regarded as an error in the corresponding  color code, commutes with a Z stabilizer generator if and only if the generator has support on a number of qubits that is a multiple of 4.
color code, commutes with a Z stabilizer generator if and only if the generator has support on a number of qubits that is a multiple of 4.
For the above 2D and 3D families of color codes we can give T explicitly. In the 2D case it is simplest to depict it, see figure 1. As for the 3D case, first notice that qubits correspond to the following elements of the triangulation of a colored tetrahedron M: all 3-simplices, the 2-simplices on the faces of M, the 1-simplices on the edges of M, and the 0-simplices that are the vertices of M. A valid choice for the set T is the union of (i) the set T3 of all 3-simplices with 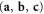 as in (18) an even permutation of the triad
as in (18) an even permutation of the triad 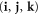 , (ii) the set T2 of all 2-simplices that are not a subsimplex of any element of T3, see figure 3, and (iii) the set T1 containing all 1-simplices that are not a subsimplex of any element of T2.
, (ii) the set T2 of all 2-simplices that are not a subsimplex of any element of T3, see figure 3, and (iii) the set T1 containing all 1-simplices that are not a subsimplex of any element of T2.
3.4. Gauge fixing
The different gauge color codes that can be defined on a given, fixed lattice are related by gauge fixing, either directly or via some other code in the family. Indeed, all the codes have a shared group 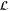 of bare logical operators (namely, those with support in all qubits) and the condition (12) is satisfied when
of bare logical operators (namely, those with support in all qubits) and the condition (12) is satisfied when 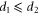 ,
, 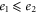 . Take e.g. D = 3. For a given geometry we might consider both the code
. Take e.g. D = 3. For a given geometry we might consider both the code 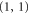 or the gauge fixed version
or the gauge fixed version  . Within
. Within 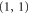 CNot and Hadamard gates are transversal. Fixing the gauge we can move into
CNot and Hadamard gates are transversal. Fixing the gauge we can move into 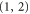 to apply transversal
to apply transversal  gates, completing a universal set of transversal gates. The ideal strategy is to transition to
gates, completing a universal set of transversal gates. The ideal strategy is to transition to  only momentarily. This way there is no need to ever measure directly the large stabilizer generators of the (1, 2) code. Instead, it is enough to measure the gauge generators in
only momentarily. This way there is no need to ever measure directly the large stabilizer generators of the (1, 2) code. Instead, it is enough to measure the gauge generators in 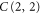 , which only involve up to 6 qubits. Notice that the only non-transversal element of the procedure is the classical computation to find the gauge operator that will fix the gauge as desired. This is entirely analogous to error correction.
, which only involve up to 6 qubits. Notice that the only non-transversal element of the procedure is the classical computation to find the gauge operator that will fix the gauge as desired. This is entirely analogous to error correction.
4. Measurements in error correction
In order to perform error correction on a stabilizer code the first step is to recover the error syndrome by measuring a collection of stabilizer operators that generate  . This can be done either directly or indirectly by performing a sequence of measurements of gauge operators from which the syndrome can be recovered [19]. In the case of CSS codes the later approach is particularly straightforward. It suffices to first measure a generating set of X-type gauge operators (which commute with each other), and then do the same with Z-type operators. The eigenvalues of X-type stabilizers can be recovered from the first set of measurements, and similarly for the Z-type and the second set.
. This can be done either directly or indirectly by performing a sequence of measurements of gauge operators from which the syndrome can be recovered [19]. In the case of CSS codes the later approach is particularly straightforward. It suffices to first measure a generating set of X-type gauge operators (which commute with each other), and then do the same with Z-type operators. The eigenvalues of X-type stabilizers can be recovered from the first set of measurements, and similarly for the Z-type and the second set.
Consider now the specific case of a (d, e) gauge color code. From the D-colex perspective in order to measure the syndrome for Z-type stabilizers we have to either measure directly stabilizer operators attached to  -cells or measure instead gauge generators attached to
-cells or measure instead gauge generators attached to 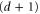 -cells. In fact, it is possible to choose to measure operators attached to d'-cells for any
-cells. In fact, it is possible to choose to measure operators attached to d'-cells for any 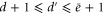 ). Whenever
). Whenever  , it is worth noting that it is not necessary to measure all the d'-cell operators. Instead, the stabilizer generator Zc on a
, it is worth noting that it is not necessary to measure all the d'-cell operators. Instead, the stabilizer generator Zc on a  -cell c can be recovered in different ways from the operators
-cell c can be recovered in different ways from the operators  , where c' stands for a d'-cell. In particular, if κ is a subset with d' elements from the set of
, where c' stands for a d'-cell. In particular, if κ is a subset with d' elements from the set of 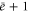 colors of c, and
colors of c, and  the set of κ-cells contained in c, then [12]
the set of κ-cells contained in c, then [12]
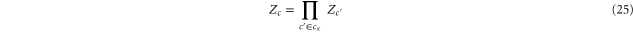
which is just a general form of (C9).
It follows that it is enough to measure at a subset of d'-cells with enough color combinations κ so that every collection of  colors has to contain one of the color combinations. E.g. for
colors has to contain one of the color combinations. E.g. for 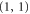 gauge color codes in 3D it suffices to choose two disjoint pair of colors, as opposed to the six possible pairs of colors.
gauge color codes in 3D it suffices to choose two disjoint pair of colors, as opposed to the six possible pairs of colors.
Consider more particularly the specific 3D lattice given in the main text. In its bulk, the 3-cells of the corresponding 3-colex have 24 qubits (0-cells) each. For a specific pair of colors, each cell has either 6 2-cells with 4 qubits each or 4 2-cells with 6 qubits each. Measuring any of the corresponding sets of 2-cell operators is enough, but measuring all of them gives redundant information that can be put to good use [25].
Coloring can be used, whatever the spatial dimension D, to organize the measurements. The idea is that cells with the same coloring have disjoint sets of vertices, and thus the related operators act on disjoint set of qubits. Then one can measure in parallel all the generators related to a color combination. Naturally, other approaches might be more optimal.
Finally, a note on error-correction itself. In CSS codes it is natural (but again not optimal) to deal separately with X and Z errors. In the case of gauge color codes this has the advantage of mapping the problem back to conventional color codes. Namely, consider a 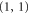 gauge color code in 3D. The error syndrome for X errors is the same as in the corresponding
gauge color code in 3D. The error syndrome for X errors is the same as in the corresponding  conventional color code, and the error syndrome for Z errors is the same as in the corresponding
conventional color code, and the error syndrome for Z errors is the same as in the corresponding 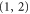 conventional color code. In the presence of measurements error, however, new scenarios open if gauge generators are measured [25].
conventional color code. In the presence of measurements error, however, new scenarios open if gauge generators are measured [25].
5. Outlook
The results presented here show that 3D gauge color codes put together some unique features (in fact, they turn out to be surprisingly resilient to errors in the error-detecting measurements, as explained in [25]). Further research would thus be desirable, e.g. regarding noise thresholds.
It is intriguing to consider quantum Hamiltonian models based on 3D gauge color codes, i.e. of the form
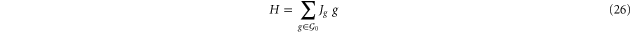
for some set 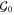 of local generators of the gauge group and couplings Jg. The fact that all the (standard) gauge generators detect fluxes [12] suggests the possibility of a self-correcting phase [26, 27].
of local generators of the gauge group and couplings Jg. The fact that all the (standard) gauge generators detect fluxes [12] suggests the possibility of a self-correcting phase [26, 27].
Topological codes have a rich behavior, but they are just part of the larger class of local codes, where spatial geometry is not relevant. This could be a path to obtain code families with the properties of 3D gauge color codes but requiring less physical qubits.
Acknowledgments
I am grateful to Stephen Bartlett, Steve Flammia and Courtney Brell for pointing out the work [16] in connection with color codes. I thank the Spanish MIC-INN grant FIS2009–10061, CAM QUITEMAD S2009-ESP-1594, the Sapere Aude grant of the Danish Council for Independent Research, the ERC Starting Grant QMULT and the CHIST-ERA project CQC. Work at Perimeter Institute is supported by Industry Canada and Ontario MRI.
Appendix A.: Transversal gates
The aim is to show that  is transversal if (3) holds, which can be written more compactly as
is transversal if (3) holds, which can be written more compactly as

by introducing the notation, for any subset 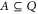
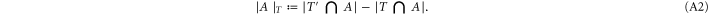
In particular, the claim is that there exists an integer k such that a logical  is implemented by applying
is implemented by applying  to qubits in T and
to qubits in T and  to the rest, i.e. in T'. The resulting gate maps, for any given subset
to the rest, i.e. in T'. The resulting gate maps, for any given subset  ,
,
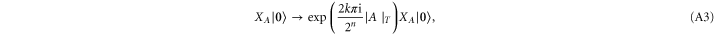
where  is the state with all physical spins up
is the state with all physical spins up 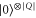 . In the code subspace the stabilizers have eigenvalue 1 and thus encoded states are superpositions of states
. In the code subspace the stabilizers have eigenvalue 1 and thus encoded states are superpositions of states  with XA a product of several X-type gauge generators and possible XQ. Taking ZQ to be the logical Z operator, an encoded state
with XA a product of several X-type gauge generators and possible XQ. Taking ZQ to be the logical Z operator, an encoded state 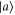 , a = 0, 1, is a superposition of states of the form
, a = 0, 1, is a superposition of states of the form
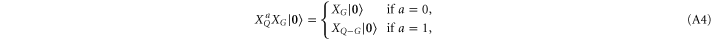
where 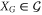 and
and  is the complement of G in Q.
is the complement of G in Q.
Suppose that, for any  , we had
, we had
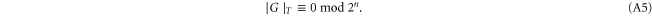
Then the states (A4) transform as follows according to (A3)
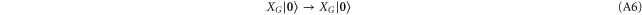
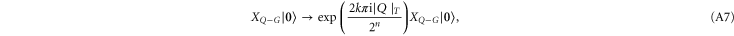
using the fact that for sets 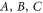 with
with 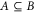

Since XQ and ZQ anticommute  is odd and so is
is odd and so is  too. Therefore
too. Therefore  and
and  are relatively prime and there exists k with
are relatively prime and there exists k with
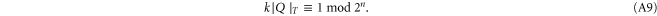
Rn is indeed implemented for such k.
Thus it suffices to show that (A5) holds. For  this is trivial. For
this is trivial. For 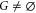 consider the stronger statement
consider the stronger statement
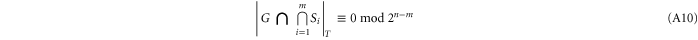
for any 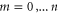 ,
,  , which reduces to (A5) for m = 0. Let
, which reduces to (A5) for m = 0. Let
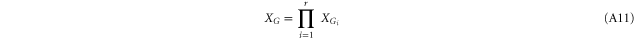
for some  . For r = 1 (A10) is true by assumption (A1). If
. For r = 1 (A10) is true by assumption (A1). If 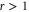 , set
, set
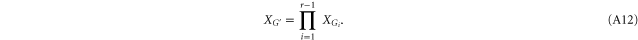
Then  , with + the symmetric difference, defined as
, with + the symmetric difference, defined as

Noticing that
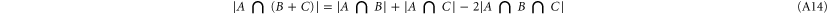
one immediately gets
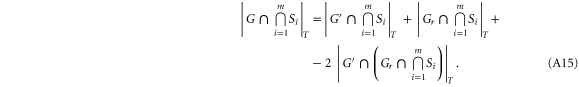
The result follows by induction on the number of generators r: all the terms on the right hand side involve less than r generators, in particular 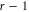 in the case of G' and 1 in the case of Gr, and
in the case of G' and 1 in the case of Gr, and

A similar result holds for k control qubits with m running from 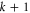 to
to  and the modulus being
and the modulus being  .
.
Appendix B.: D-colexes and duality
D-colexes are D-dimensional lattices with certain colorability properties. For a lattice here it is meant a division of a closed D-manifold into D-cells, which are themselves composed of 0-cells (vertices), 1-cells (edges) and so on in the usual way. The colorability reads:
Every d-cell is labeled by d colors from a given set of  colors. Given a cell c with color set κ, the cells with c as a subcell are in one to one correspondence (according to their label) with the color sets with κ as a subset.
colors. Given a cell c with color set κ, the cells with c as a subcell are in one to one correspondence (according to their label) with the color sets with κ as a subset.
E.g. at every vertex 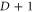 edges meet, each with a different color. Notice that every two cells with the same color set must be equal or disjoint. This in turn implies that all 2-cells have an even number of edges: the edges along the boundary of the 2-cell must have alternate colors. Another easy property is that the boundary of every d-cell is itself a
edges meet, each with a different color. Notice that every two cells with the same color set must be equal or disjoint. This in turn implies that all 2-cells have an even number of edges: the edges along the boundary of the 2-cell must have alternate colors. Another easy property is that the boundary of every d-cell is itself a 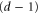 -colex (in particular a
-colex (in particular a  -sphere).
-sphere).
The dual of a D-colex is a simplicial lattice with the vertex colorability properties given in the text, in particular, the dual of a d-cell with color set κ is labeled with the color set 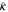 , defined as the complement of κ in the set of
, defined as the complement of κ in the set of 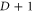 colors. Recall that under duality d-cells are mapped to
colors. Recall that under duality d-cells are mapped to  -cells in such a way that the relationship 'is a subcell of' is reverted. It is then easy to check that one indeed gets a simplicial lattice with the right coloring: each of the subsimplices of a given simplex with color set κ is labeled by a nonempty subset of κ.
-cells in such a way that the relationship 'is a subcell of' is reverted. It is then easy to check that one indeed gets a simplicial lattice with the right coloring: each of the subsimplices of a given simplex with color set κ is labeled by a nonempty subset of κ.
The above applies to closed D-manifolds, but of interest here are punctured D-colexes. They are obtained by starting from a D-colex on a D-sphere and removing a single vertex, together with all the cells that contain it. In the dual simplicial lattice this means removing a D-simplex together with all of its subsimplices, giving rise to the simplex collections 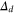 of the main text. But some of the simplices in
of the main text. But some of the simplices in  have subsimplices that are not in any of the
have subsimplices that are not in any of the 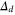 . The simplicial lattice M of the main text is recovered by keeping instead only those simplices that still retain all their subsimplices.
. The simplicial lattice M of the main text is recovered by keeping instead only those simplices that still retain all their subsimplices.
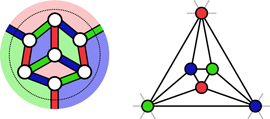
All this is illustrated for D = 2 in figure B1
, where M (shaded), its closed spherical version and the corresponding punctured colex are compared. It is apparent that the colex picture allows a more easy visualization of the code, with qubits placed at vertices. Notice that in the D-colex perspective the generators of stabilizer and gauge group correspond to the following geometrical objects: for a (d, e) gauge color code X stabilizer generators are 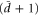 -cells, Z stabilizer generators are
-cells, Z stabilizer generators are  -cells, X gauge generators are
-cells, X gauge generators are  -cells and Z gauge generators are
-cells and Z gauge generators are 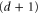 -cells.
-cells.
Figure B1. A punctured 2-colex with faces colored with their complementary/dual color (right) compared to the corresponding triangulation of a sphere (left). Duality is explicit in the central figure.
Download figure:
Standard image High-resolution imageAnother interesting point is that when we describe color codes by giving M it is obvious what the simplest example is: that in which the triangulation of M is composed of just a single D-simplex that coincides with M itself. From the colex perspective this corresponds to a punctured D-colex that, without the vertex removed, is the boundary of a 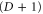 -cube. It suffices to color parallel edges of the cube with the same color to get the colex structure, which is thus a D-sphere as required. The dual simplicial picture of this simplest case serves as a pattern for the combinatorial prescription given in the main text for 'closing' a generic triangulation of M by adding extra simplices: it shows that the prescription indeed produces a D-sphere as stated.
-cube. It suffices to color parallel edges of the cube with the same color to get the colex structure, which is thus a D-sphere as required. The dual simplicial picture of this simplest case serves as a pattern for the combinatorial prescription given in the main text for 'closing' a generic triangulation of M by adding extra simplices: it shows that the prescription indeed produces a D-sphere as stated.
Appendix C.: Perfect colexes
C.1. Motivation
The result (24) was already proven in [14] for conventional color codes in the special case 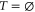 , i.e. when the same rotation is applied to all physical qubits. Generalizing slightly to the subsystem case, this means that the result hinged on gauge generators
, i.e. when the same rotation is applied to all physical qubits. Generalizing slightly to the subsystem case, this means that the result hinged on gauge generators 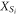 satisfying
satisfying

These are local constraints on the D-colex, because the generators 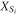 are local.
are local.
Fortunately conditions (C1) can be simplified a lot. This is due to the following property of colexes [14]:
Given a collection of cells  with color sets
with color sets 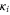 , their intersection is a collection of cells with color label κ:
, their intersection is a collection of cells with color label κ:
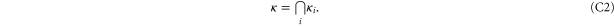
The cells of the collection must be disjoint, because they have the same color. Of interest here is the case where the m cells ci have all dimension  (as they correspond to gauge generators). Suppose that
(as they correspond to gauge generators). Suppose that  , so that the cells in the intersection have dimension d'. Since there are a total of
, so that the cells in the intersection have dimension d'. Since there are a total of 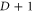 colors, m cannot have an arbitrary value, but rather
colors, m cannot have an arbitrary value, but rather
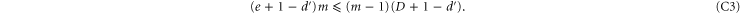
Indeed, consider the collection of pairs (i, r) where 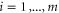 and r is one of the colors in
and r is one of the colors in  . The left hand side is the total number of such pairs. The right hand side is the maximum number of pairs that we could form with such r, which cannot be shared by the m cells ci and therefore can appear at most
. The left hand side is the total number of such pairs. The right hand side is the maximum number of pairs that we could form with such r, which cannot be shared by the m cells ci and therefore can appear at most  times. Thus the inequality, which can be restated as
times. Thus the inequality, which can be restated as
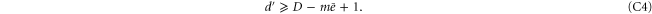
Going back to (C1), the case m = n will be satisfied if and only if the intersection is composed of cells of dimension at least 1. For this to hold, the right hand side of (C4) hast to be greater or equal than one, i.e. the inequality (24) must hold. Naturally, the conditions (C1) must be satisfied also for  , but when (24) holds
, but when (24) holds
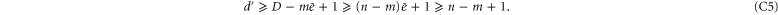
Thus the conditions (C1) are satisfied if all d-cells have a number of vertices multiple of  . This motivates the following definition:
. This motivates the following definition:
A D-colex is perfect if every cell has  vertices, where d is the dimension of the cell.
vertices, where d is the dimension of the cell.
In [14] the problem of the existence of perfect colexes was left open. The rest of this appendix is devoted to show that one can always obtain a perfect colex from a generic one by transforming the lattice in the neighborhood of a collection of vertices T. The next appendix in turn shows that the actual substitution of the generic colex by its perfect counterpart is unnecessary. Rather, a transversal 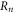 is recovered by inverting the rotation at those qubits in the set T.
is recovered by inverting the rotation at those qubits in the set T.
C.2. Construction
Crucial to the construction of perfect colexes are the 'simplest' colexes described in the previous appendix, which are perfect. Recall that the boundary of a 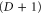 -hypercube is a D-colex, from which the 'simplest' punctured D-colex is obtained by removing a vertex and all the cells containing it. Here instead we consider removing a small neighborhood of a vertex, so that we are left with a D-dimensional ball that contains
-hypercube is a D-colex, from which the 'simplest' punctured D-colex is obtained by removing a vertex and all the cells containing it. Here instead we consider removing a small neighborhood of a vertex, so that we are left with a D-dimensional ball that contains  vertices and in which all 'complete' d cells have
vertices and in which all 'complete' d cells have  vertices, while the 'incomplete' ones are lacking the removed vertex and thus keep only
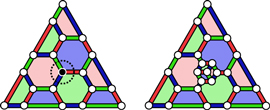
vertices, while the 'incomplete' ones are lacking the removed vertex and thus keep only  . The procedure to make a generic colex into a perfect one involves substituting the neighborhood of each of the vertices in a collection T by such D-balls, as in figure C1
(this is actually a connected sum with a neat combinatorial description, see [12]). Therefore, a given d cell c with vertex set Vc in the original colex gives rise to a d-cell c' with vertex set
. The procedure to make a generic colex into a perfect one involves substituting the neighborhood of each of the vertices in a collection T by such D-balls, as in figure C1
(this is actually a connected sum with a neat combinatorial description, see [12]). Therefore, a given d cell c with vertex set Vc in the original colex gives rise to a d-cell c' with vertex set  such that
such that
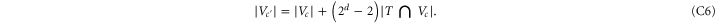
All the new d-cells added to the colex have 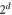 vertices.
vertices.
Figure C1. Direct and dual pictures of the transformation applied to each element of T for D = 2. In the 2-colex picture (left) the neighborhood of each vertex in T is substituted by a 2-ball (with contour the dotted line) obtained from a colored cube by removing the neighborhood of a vertex. In the simplicial picture (right) each 2-simplex in T is divided in 7 pieces. This adds 3 new vertices (those in the interior), each of a different color.
Download figure:
Standard image High-resolution imageThe procedure is generically valid only for either spherical D-colexes, which yield trivial codes, or punctured D-colexes, which are the ones of interest here. Notice however that in order for the above to make sense we need to be sure that every vertex has the right kind of neighborhood, which is true in the bulk but not on the boundary for the punctured colex. Fortunately this is not an important issue. Indeed, it suffices to make the colex into an spherical one by adding a 0-cell in the usual way, perform the changes at T vertices, and then remove the added vertex together with all the cells that contain it.
It might be worth describing the dual picture, in which T is a set of D-simplices. Each simplex in T is divided in 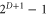 pieces: there are
pieces: there are  new vertices, and for each color set κ with
new vertices, and for each color set κ with 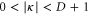 there is a new D-simplex that has as vertices (i) the new vertices with labels κ and (ii) the vertices of the removed simplex with colors in
there is a new D-simplex that has as vertices (i) the new vertices with labels κ and (ii) the vertices of the removed simplex with colors in 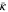 . Figure C1 illustrates the dual picture for D = 2. Notice that this is nothing but the construction that was already used in the main text to 'close' M to form a sphere.
. Figure C1 illustrates the dual picture for D = 2. Notice that this is nothing but the construction that was already used in the main text to 'close' M to form a sphere.
It is convenient to first consider the D = 2 case. Figure C2
illustrates the procedure in a 2-colex for which T is composed of a single element, i.e. it is enough to substitute the neighborhood of a single vertex. In the original 2-colex there are 3 faces that have 6 vertices. In the transformed one, each of these faces has gained 2 vertices, so that they have  vertices, as required.
vertices, as required.
Figure C2. A punctured 2-colex (left) and a perfect punctured 2-colex (right) obtained from the former by changing the lattice in the neighborhood of the vertex marked in black.
Download figure:
Standard image High-resolution imageIt is apparent what the T set of vertices should satisfy in the D = 2 case. 'Good' faces, those with  vertices, must have an even number v of vertices in T, so that they end up with
vertices, must have an even number v of vertices in T, so that they end up with
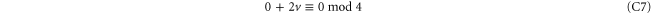
vertices and stay good. 'Bad' faces, those with 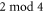 vertices, must have an odd number v of vertices in T, so that they end up with
vertices, must have an odd number v of vertices in T, so that they end up with
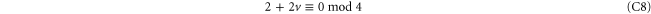
vertices and become good. But, is there always such a set T? How do we find it?
At this point it is useful to consider a different problem: error correction. In the D = 2 case, there is a single color code:  . Its stabilizer generators are attached to faces. Given an error of the form XT, where T is some set of qubits/vertices, its syndrome amounts to the collection of faces f such that XT and Zf anticommute, where Zf stands for the Z stabilizer at f. These syndrome faces f are simply those that contain an odd number of vertices from the set T. I.e. the syndrome of XT corresponds to those faces that gain
. Its stabilizer generators are attached to faces. Given an error of the form XT, where T is some set of qubits/vertices, its syndrome amounts to the collection of faces f such that XT and Zf anticommute, where Zf stands for the Z stabilizer at f. These syndrome faces f are simply those that contain an odd number of vertices from the set T. I.e. the syndrome of XT corresponds to those faces that gain  vertices when the vertices of T are subject to the above geometrical transformation. This allows to answer both questions above. Regarding existence, in the case of punctured
vertices when the vertices of T are subject to the above geometrical transformation. This allows to answer both questions above. Regarding existence, in the case of punctured  color codes all syndrome sets are possible [10]. To find T it suffices to solve a set of binary linear equations, just the same way that one can find the possible errors from the error-syndrome.
color codes all syndrome sets are possible [10]. To find T it suffices to solve a set of binary linear equations, just the same way that one can find the possible errors from the error-syndrome.
The stage is set to show how the construction works for general punctured D-colexes. There are two steps to this. First, it is possible to find a set of vertices T such that the resulting D-colex has only good 2-cells as above. Second, it turns out that the resulting colex is perfect.
The existence of T is not obvious. The reason for this is that for 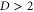 not every syndrome is possible. In particular, consider the
not every syndrome is possible. In particular, consider the  conventional color code on the given D-colex: it has Z stabilizer generators attached to 2-cells. These 2-cell operators Zf are not independent. Instead, in a punctured code they satisfy only local constraints that have their origin in the structure of 3-cells [12]. Namely, every 3-cell c has 3 colors, and thus is composed of 2-cells with 3 different color sets
conventional color code on the given D-colex: it has Z stabilizer generators attached to 2-cells. These 2-cell operators Zf are not independent. Instead, in a punctured code they satisfy only local constraints that have their origin in the structure of 3-cells [12]. Namely, every 3-cell c has 3 colors, and thus is composed of 2-cells with 3 different color sets 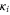 ,
,  . Let
. Let 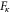 be the set of 2-cells of c with color κ, where
be the set of 2-cells of c with color κ, where  . By definition, every vertex of c belongs exactly to one 2-cell in each
. By definition, every vertex of c belongs exactly to one 2-cell in each 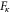 . A trivial consequence is
. A trivial consequence is
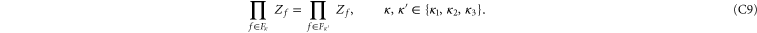
In words: the number of 2-cell operators Zf with 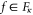 and negative syndrome is even if and only if the number of 2-cell operators Zf with
and negative syndrome is even if and only if the number of 2-cell operators Zf with 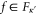 and negative syndrome is even.
and negative syndrome is even.
Fortunately, the constraints (C9) are no obstacle for the present purpose because bad 2-cells satisfy them. Namely, if among the 2-cells in 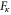 there are
there are  bad ones, then
bad ones, then 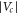 , the total number of 0-cells in c, satisfies
, the total number of 0-cells in c, satisfies
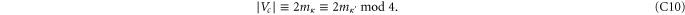
This follows again from the fact that every vertex of c belongs exactly to one 2-cell in each of the sets  : the elements of Vc can be counted by adding the number of vertices in each 2-cell of
: the elements of Vc can be counted by adding the number of vertices in each 2-cell of 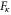 for any given κ: the result is always the same, but (C10) yields
for any given κ: the result is always the same, but (C10) yields
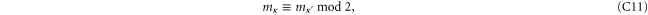
which means that a syndrome such that 2-cells have eigenvalue 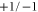 if they are respectively good/bad does exist because the constraints (C9) are satisfied. There exists T such that the set of 2-cell operators Zf that anticommute with XT is the same as the set of bad 2-cells.
if they are respectively good/bad does exist because the constraints (C9) are satisfied. There exists T such that the set of 2-cell operators Zf that anticommute with XT is the same as the set of bad 2-cells.
It only remains to show that every D-colex with no bad 2-cells is perfect. This can be done recursively. Assume that for a given D-colex with no bad 2-cells every d-cell has 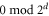 vertices. The aim is to show that every
vertices. The aim is to show that every 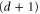 -cells has
-cells has  vertices.
vertices.
First, every such 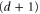 -cell has a boundary that is a spherical d-colex. From this spherical d-colex one can build a new 'shrunk' d-dimensional lattice by shrinking all the d-cells with a given color set κ to a point [12]:
-cell has a boundary that is a spherical d-colex. From this spherical d-colex one can build a new 'shrunk' d-dimensional lattice by shrinking all the d-cells with a given color set κ to a point [12]:
- The 0-cells of the shrunk lattice correspond to d-cells with color set κ, or κ-cells, of the d-colex.
- The 1-cells of the shrunk lattice correspond to 1-cells of color r of the d-colex, or r-cells, where r is the only color with
 .
. - The 2-cells of the shrunk lattice correspond to 2-cells with color sets
 such that
such that  . In particular, the shrunk 2-cell has an even number of 1-cells in its boundary because the original 2-cell has
. In particular, the shrunk 2-cell has an even number of 1-cells in its boundary because the original 2-cell has  1-cells and half of them are r-cells.
1-cells and half of them are r-cells.
Since the homology of the sphere is trivial and all the 2-cells have an even number of edges, the graph formed by the 0-cells and 1-cells of the shrunk lattice is bipartite. Indeed, any closed path must have an even number of vertices and edges because (i) as a 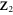 1-chain it has no boundary and thus must be a sum of boundaries of 2-cells and (ii) the sum of 1-chains with an even number of edges must have an even number of edges.
1-chain it has no boundary and thus must be a sum of boundaries of 2-cells and (ii) the sum of 1-chains with an even number of edges must have an even number of edges.
Therefore, the set of κ-cells in the d-colex is the disjoint union of two sets A and B, and every r-cell in the d-colex shares a vertex with exactly one element of the set A. Since every κ-cell in A has  vertices, it follows that there are
vertices, it follows that there are 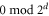 r-cells and therefore
r-cells and therefore 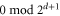 vertices in the d-colex (every vertex belongs exactly to one r-cell, and each r-cell has 2 vertices).
vertices in the d-colex (every vertex belongs exactly to one r-cell, and each r-cell has 2 vertices).
Appendix D.: Transversal Rn in color codes
According to the discussion in appendix C.1, if condition (24) is satisfied then 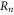 can be implemented transversally in a (d, e) gauge color code as long as the colex satisfies the following property for some set T of vertices
can be implemented transversally in a (d, e) gauge color code as long as the colex satisfies the following property for some set T of vertices
Every d-cell c of the D-colex has a set Vc of vertices with
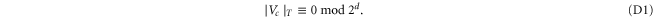
indeed, (A1) can be recovered from (D1) using the fact that for  disjoint sets
disjoint sets
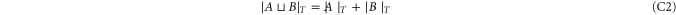
The trick to get (D1) is to choose T as in the previous appendix. Indeed, one can compare the given colex with the perfect one obtained by transforming the neighborhoods of the T vertices. The cell c maps to a new cell c' in the perfect colex and, in the notation of (C6),
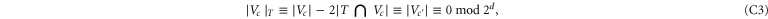
where the first equivalence is by definition of  , the second follows from (C6), and the third is due to the perfection of the final colex.
, the second follows from (C6), and the third is due to the perfection of the final colex.